Finally I have some time left to update this website. I would like to proceed with another part into the introduction to the four dimensional complex numbers. The previous part 20 dates back to 02 Feb 2019 and that too was about this structure of the non-invertible numbers in four dimensional complex space.

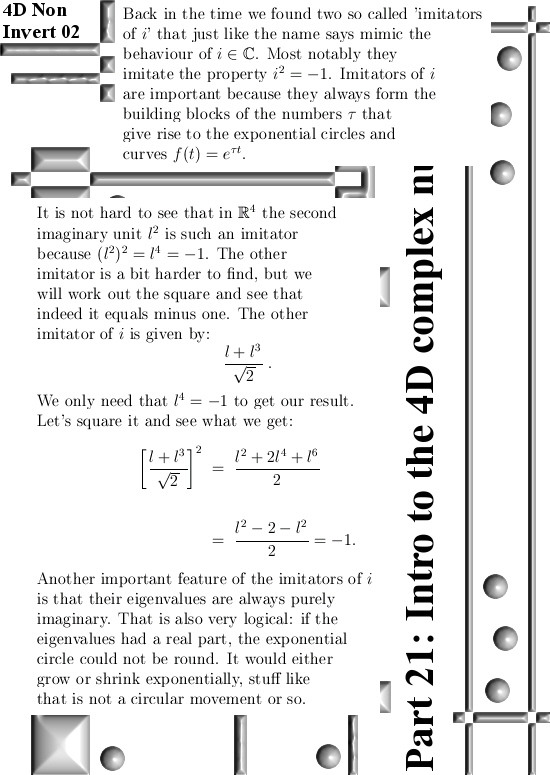
When I was reading back a few of my own old writings like part 20 from 02 Feb it struck me that those non-invertible numbers are all just linear combinations of the so called imitators of i. Imitators of i live always in dimensions higher than 2 and they mimic the behaviour of the number i from the complex plane. For example in the spaces of the 3D complex and circular numbers those imitators are not capable of squaring to minus one but they do a pretty good job at rotating stuff by 90 degrees if you multiply by them. In the 4D complex space there are two of such imitators and they do square up to minus one. Of course this is related to the fact you can find two copies of the 2D complex plane in the 4D complex space. So in that regard the 4D complex numbers are a bit different compared to the 4D quaternions that exist of 3 copies of the complex plane (but those do not commute and as such you cannot differentiate or integrate stuff).

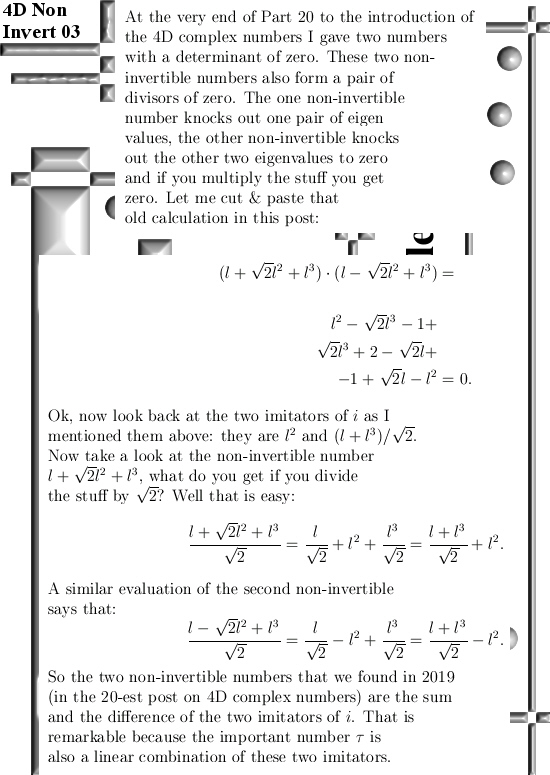
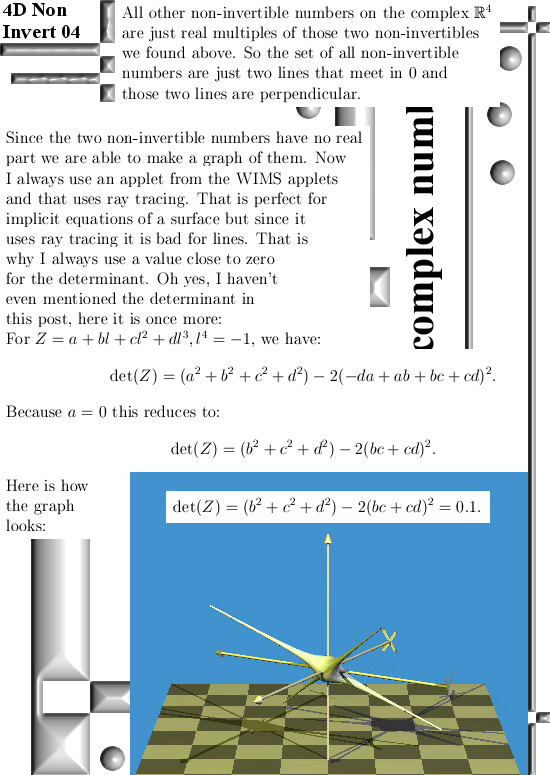
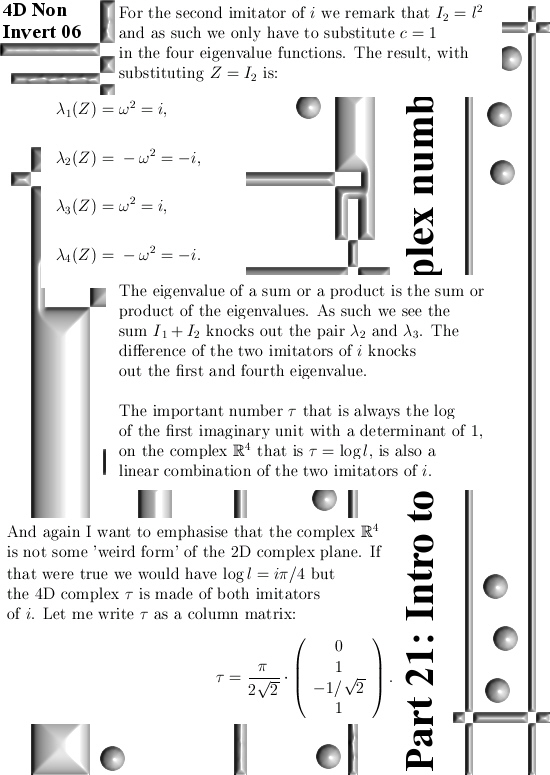
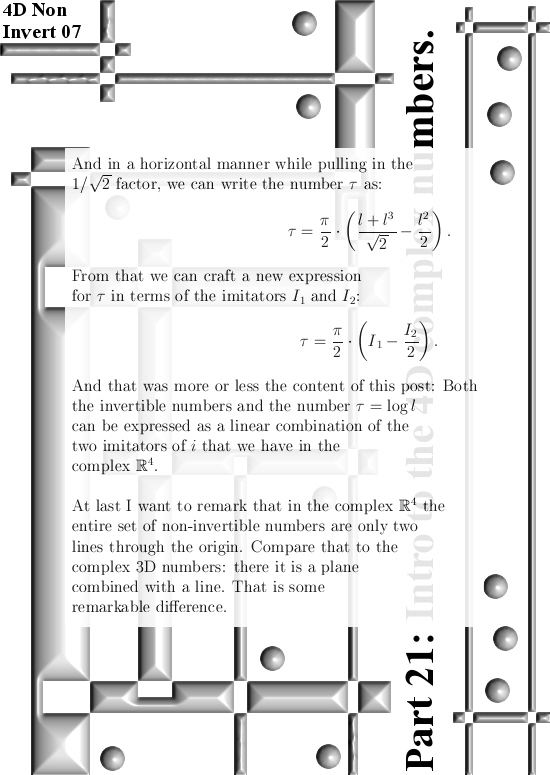
Another interesting detail is that in the 4D complex number system the set on non-invertible numbers consists of just two lines that are perpendicular to each other. That is very different from the 3D situation where the set of non-invertibles is always a plane combined with a perpendicular line through zero. The reason that in 4D complex space the set is so small lies of course in the matrix representation and the determinant. On the 4D complex space the determinant is non-negative, just like the determinant is non-negative on the complex plane. Every 4D complex number has four eigenvalues and they come in conjugate pairs, so the product of these four eigenvalues gives the determinant hence the determinant cannot be a negative real number.

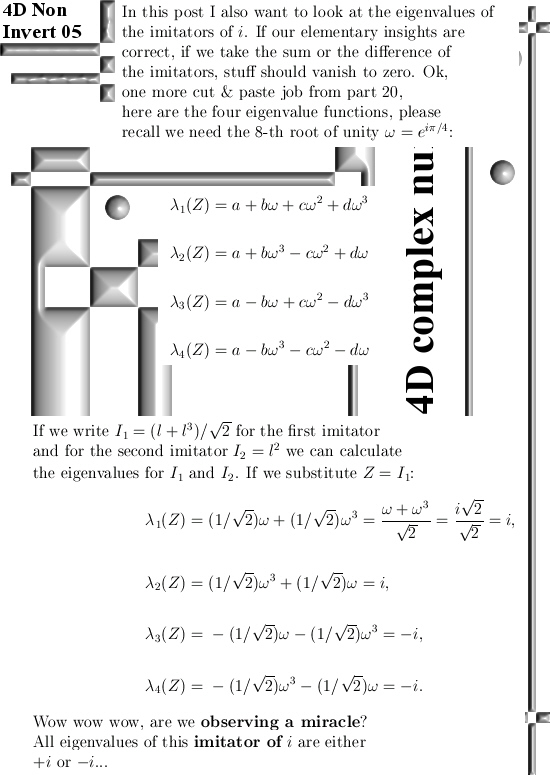
And say for yourself: aren’t the eigenvalue functions a very handy thing? If you want to find the eigenvalues of let’s say the 4D complex number Z = 1 + 2l + 3l^2 + 4l^3, that is often a horrible mathematical exercise. But once you have these four eigenvalue functions, you simply plug in any Z and voila: there are your 4 eigenvalues.
This post is seven pictures long, as usual in the 550×775 pixel size.

Ok, that was it for this post. Till updates my dear reader.