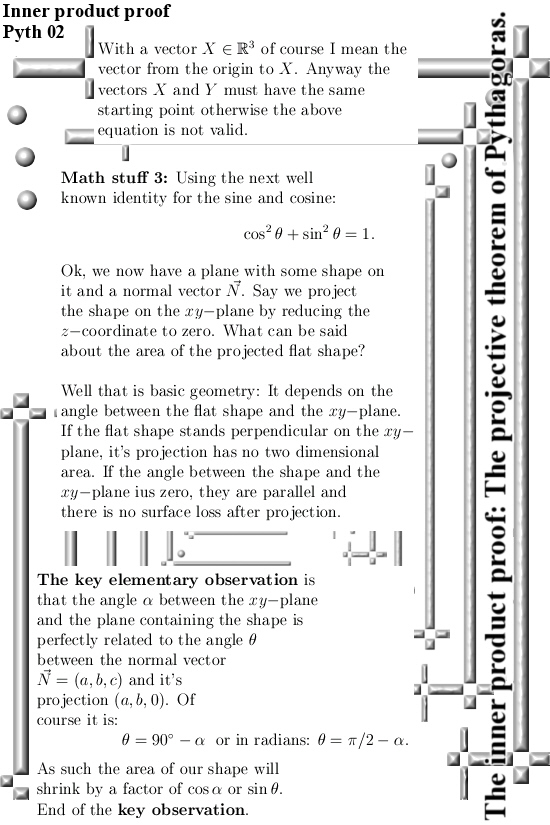
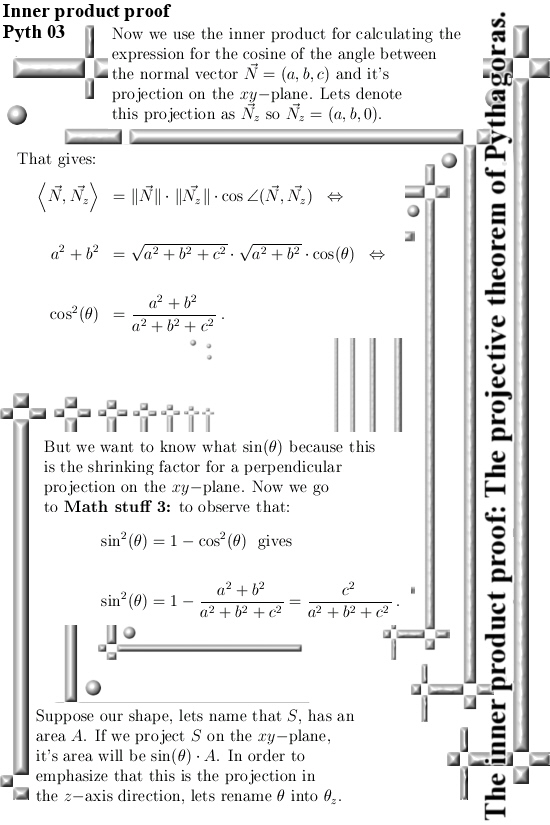
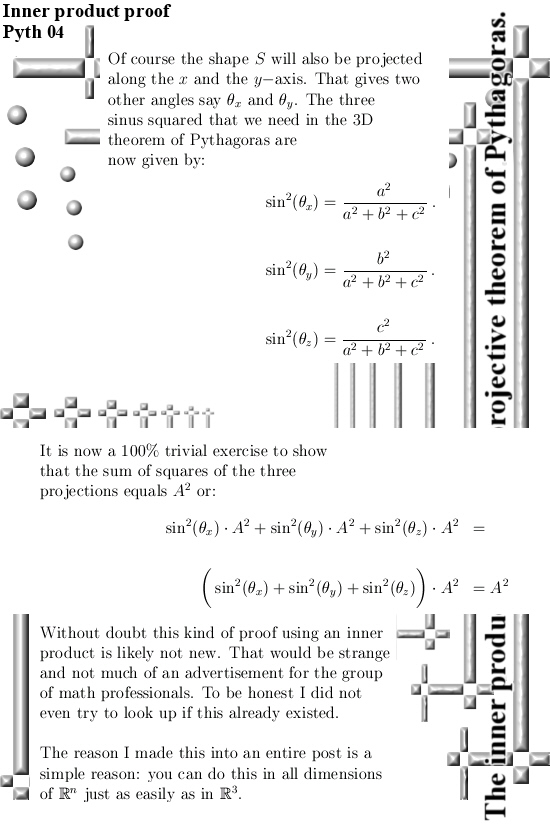
In this post we look in detail at a very simple yet important polynomial namely
p(X) = X (X – 1).
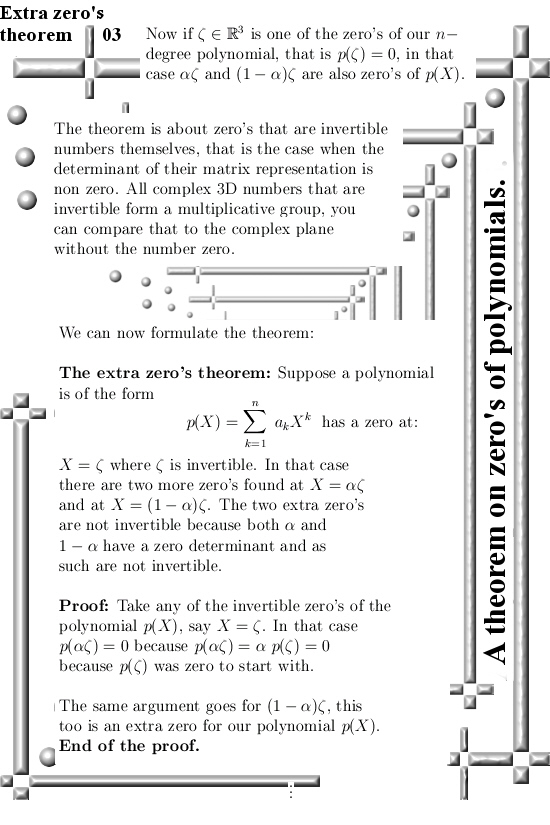
Why does it have four zero’s in the space of 3D complex numbers? Well if you solve for the zero’s of p so try to solve p(X) = 0, that is you are looking for all numbers such that X^2 = X.
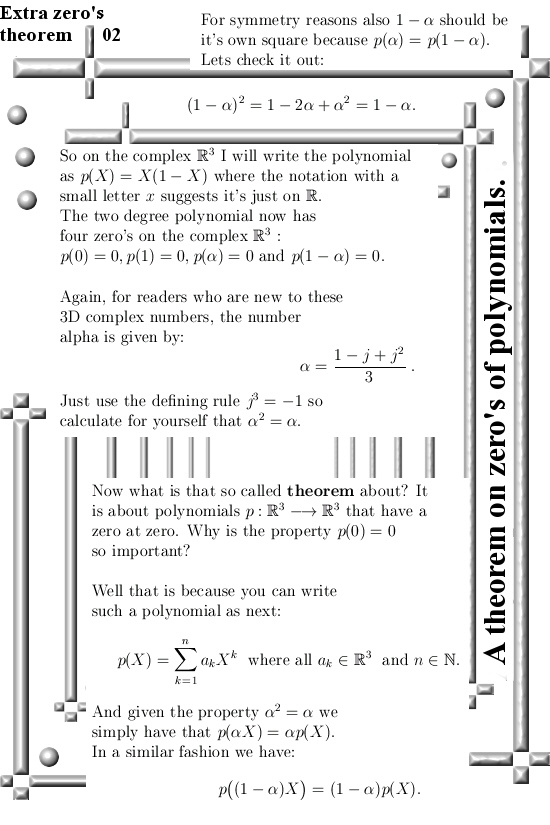
These numbers are their own square, on the real line or on the complex plane there are only two numbers that are their own square namely 0 and 1.
On the space of 3D complex numbers we also have an exponential circle and the midpoint of that circle is the famous number alpha. It is a cakewalk to calculate that alpha is it’s own square just like (1 – alpha).
This post is four pictures long in the size 550×825 pixels so it is not such a long read this time. In case you are not familiar with this number alpha, use the search function on this website and search for the post “Seven properties of the number alpha”. Of course since it is math you will also need a few days time of thinking the stuff out, after all the human brain is not very good at mathematics…
Well have fun reading it.
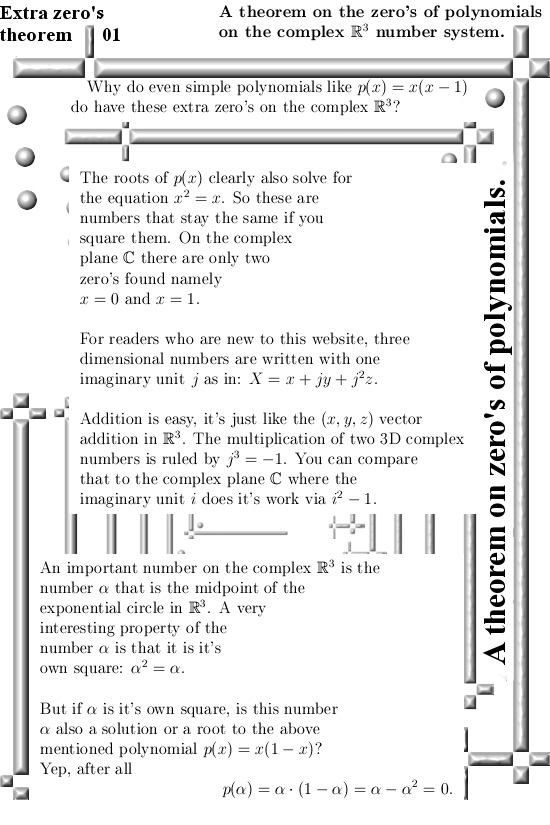

The last crazy calculation shows that a polynomial in it’s factor representation is not unique. Those zero’s at zeta one and zeta two are clearly different from 0 and 1 but they give rise to the same polynomial.
At last I want to remark that unlike on the complex plane there is no clean cut way to tell how many zero’s a given polymial will have. On the complex plane it is standard knowledge that an n-degree polynomial always has n roots (although these roots can all have the same value). But on the complex 3D numbers it is more like the situation on the real line. On the real line the polynomial p(x) = x^2 + 1 has no solution just like it has no solution on the space of 3D complex numbers.
That was it for this post, thanks for your attention.