Monthly Archives: July 2018

On a possible model for solar loops: rotating plasma.

4D complex numbers, Magnetism, Matrix representation

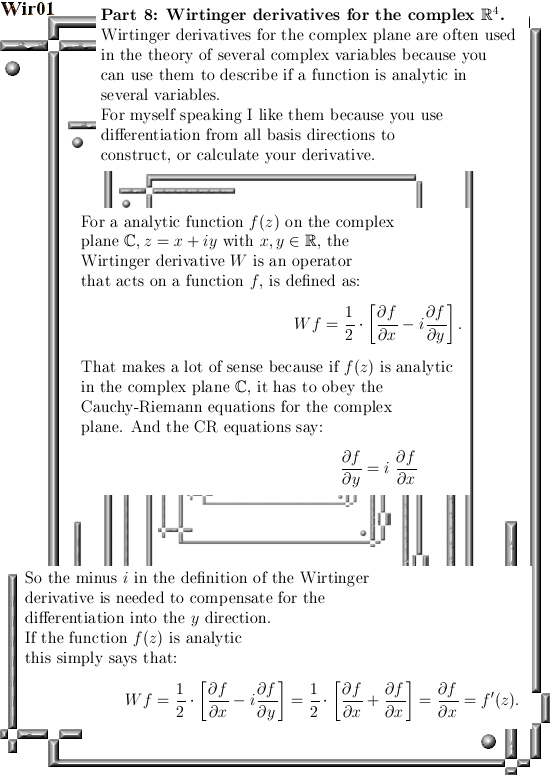
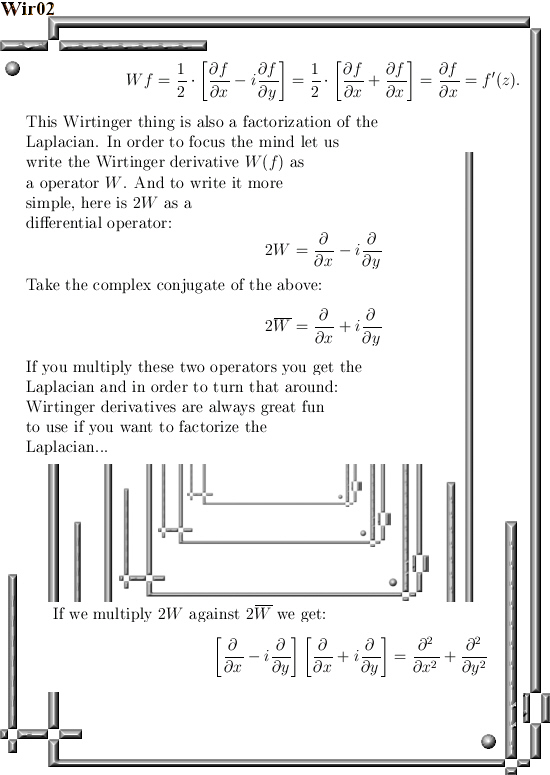
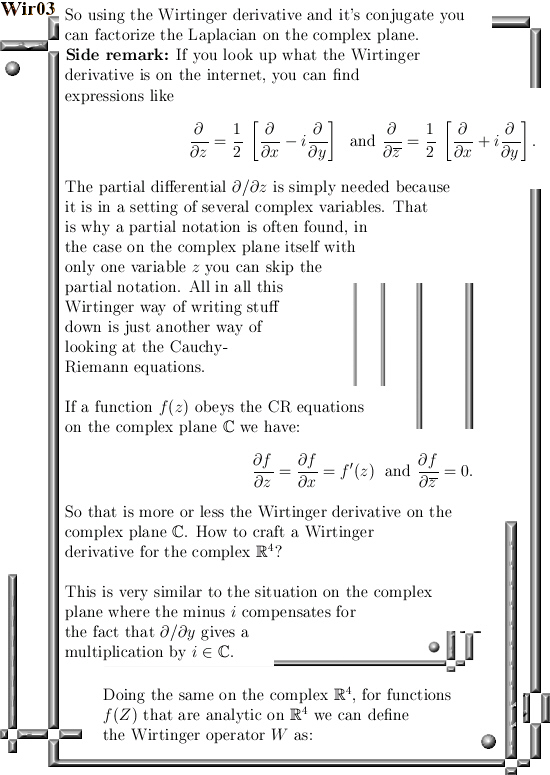
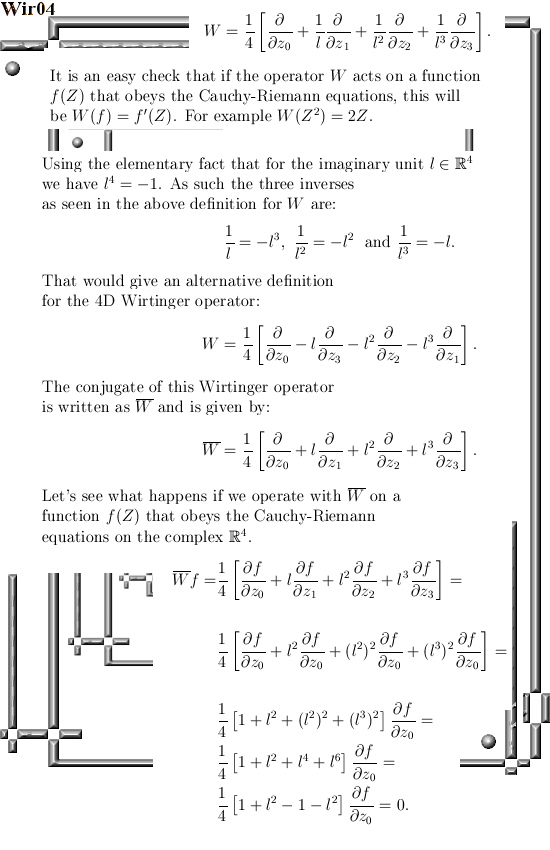
Yes that is all there is: spinning plasma… At the end of last year’s summer I had figured out that if indeed electrons have far more acceleration compared to the protons, if on the sun the solar plasma starts rotating this caused a lot of electrons flying out and as such the spinning plasma would always be electrically positive.

But at the time I had no clue whatsoever about why there would be spinning plasma at the surface of the sun but lately I found the perfect culprit: The sun spins much faster at the equator compared to the polar regions.

This spinning plasma is visible at the surface of the sun as the famous sun spots and it is known these sun spots are places of strong magnetic fields.

There is a bit of a weak spot in my simple model that says all spinning plasma creates a strong magnetic field because if the solar spots are at there minimum none of them are observed for a relatively long time. The weak spot is: Why would there be no tornado like structures be made during this minimum of solar spots? After all the speed difference is still there between the equator regions and the polar regions.

Anyway the good thing is that my simple model is very falsifiable: If you can find only one spinning tube-shaped or tornado-shaped plasma structure that not makes magnetic fields, the simple model can be thrown into the garbage bin.

The simple model is found in Reason number 65 as why electrons cannot be magnetic dipoles on the other website:

Reason 65: A possible model for solar loops going between two solar spots.
http://kinkytshirts.nl/rootdirectory/just_some_math/monopole_magnetic_stuff03.htm#22July2018

The main feature of the solar loops is that before your very own eyes you see all that solar plasma accelerating while according to the standard model of physics this is not possible.

Now there are plenty of physics professors stating that electrons can be accelerated by a magnetic field but if you hear them saying that you know they have never done the calculations that make it at least plausible that non constant magnetic fields are the main driver of electron acceleration.

Here are two nice pictures of what I am trying to explain with my simple model.

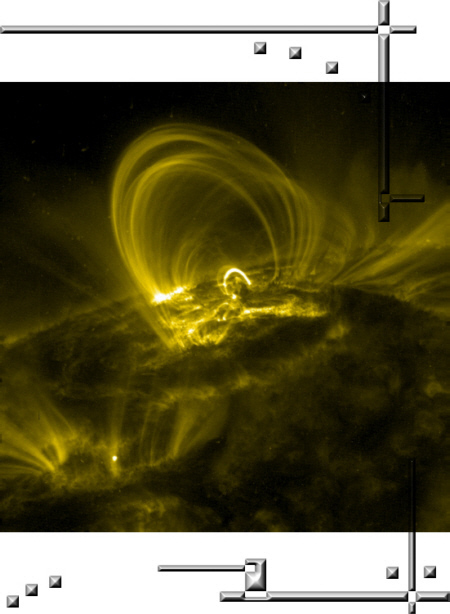
The above picture is in the UV part of the spectrum.

After having said that, the next post is like planned about numerical evaluations related to the four coordinate functions of the new found exponential curve f(t) for the 4D complex numbers. I hope to finish it later this week.

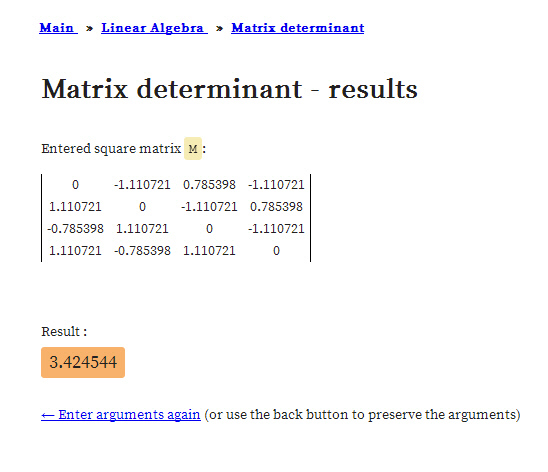
Now we are talking about cute numerical results anyway, in the next picture you can see numerical validation that the number tau in the 4D complex space is invertible because the determinant of it’s matrix representation is clearly non-zero.

You might say ‘so what?’.  But if the number tau is invertible on the 4D complex numbers (just like the complex plane i has an inverse) in that case you can also craft a new Cauchy integral representation for that!

Again you might say ‘so what?’. But Cauchy integral representation is highly magical inside complex analysis related to the complex plane. There is a wiki upon it but the main result is a bit hard to swallow if you see it for the first time, furthermore the proof given is completely horrible let alone the bullshit after that. Anyway here it is, proud 21-century math wiki style:

Cauchy’s integral formula
https://en.wikipedia.org/wiki/Cauchy%27s_integral_formula

Ok, let’s leave it with that. Till updates my dear reader.

Part 8 of the 4D basics: Wirtinger derivatives.

4D complex numbers, CR equations, Laplacian

This is the 91-th post on this website so surely but slowly this website is growing on. This post was more or less written just for myself; I don’t know if the concept of Wirtinger derivates is used a lot in standard complex analysis but I sure like it so that’s why we take a look at it.

The idea of a Wirtinger derivative is very simple to understand: You have some function f(Z) and by differentiating it in the direction of all four basis vectors you craft the derivative f'(Z) from that.

At the basis for all the calculations we do in this post are the Cauchy-Riemann equations that allow you to rewrite the partial derivatives we put into the Wirtinger derivative.

The main result in this post is as follows:
We take our Wirtinger operator W and we multiply it with the 4D complex conjugate of W and we show that this is a real multiple of the Laplacian.

The 4D case is more or less the same as on the complex plane, that is not a miracle because in previous 4D basics we already observed two planes inside the 4D complex numbers that are isomorphic to the complex plane. So it is not much of a surprise the entire 4D space of complex numbers behaves in that way too; all functions are harmonic that is the Laplacian of such a function is zero.

This post is ten pictures long, most of them are size 550 x 775 but a few of them are a bit broader like 600 x 775 because the calculations are rather wide.

On the scale of things this post is not ultra important or so, it is more like I wrote it for myself and I wanted to look in how much this all was different from the three dimensional case.

Here are the pics:

Further reading from a wiki (of course that is only about 2D complex numbers from the complex plane):
Wirtinger derivatives
https://en.wikipedia.org/wiki/Wirtinger_derivatives

Ok, that was it. Till updates.