Yesterday I was editing the six pictures for this update and all of a sudden I realized I had made a dumb dumb mistake: The pictures count down from number 7 to number 2…

I had processed them in the wrong order; I had made seven background pictures but I filled in the math text in the wrong order.

All in all I decided to leave it this way; it might be a stupid mistake but it is not a critical mistake like making a critical math error or having wrong ideas about what is actually happening on the math level. It is just an editing error and also funny. So I leave it this way.

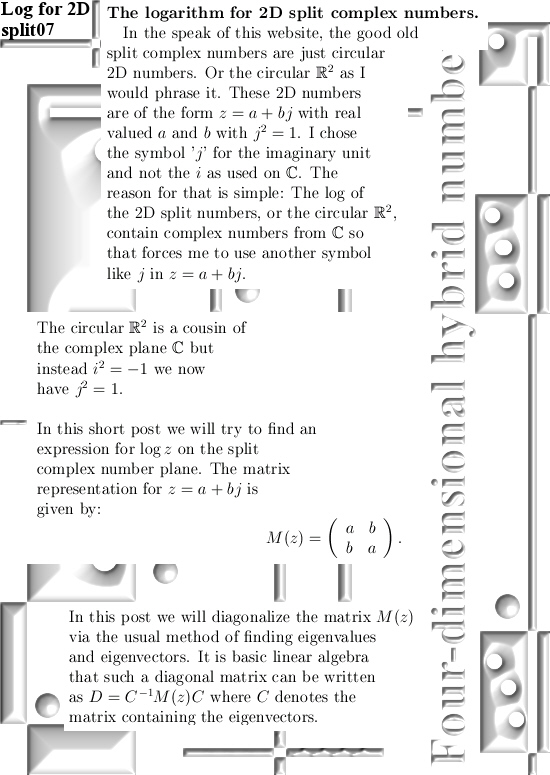
In this post we look at the so called split complex numbers, they are the cousin of the numbers from the complex plane. The only difference is that where in the complex plane the square of the imaginary unit equals minus one, for the split complex numbers this equals plus one.

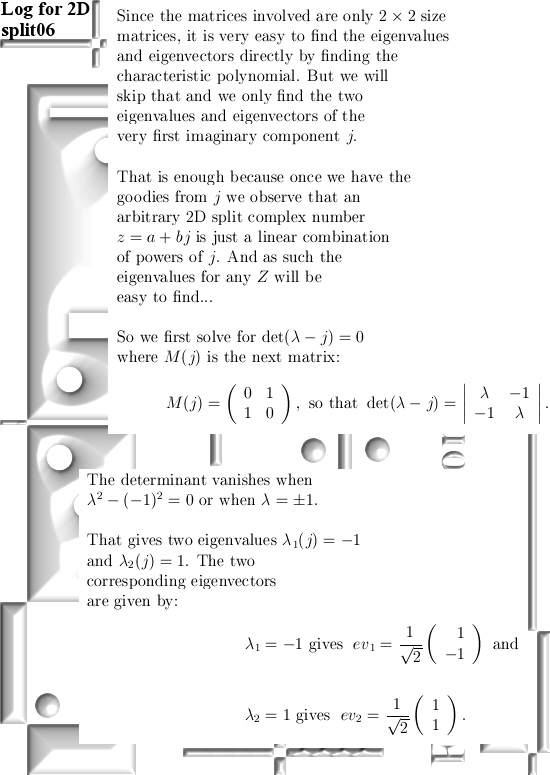
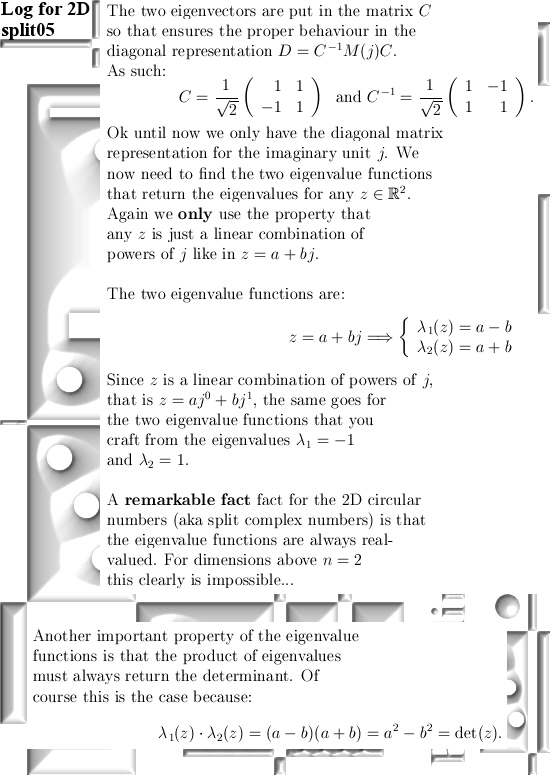
Although this is a minor change, split complex numbers are not a field because it contains non-invertible numbers outside the number 0. All I do in this post is finding the eigenvalues and eigenvectors of all split complex numbers and via taking the log of the eigenvalues we calculate what the log of an arbitrary split complex number is.

In the speak of this website the split complex numbers are just the 2D circular numbers. Remeber in all dimensions numbers are complex or circular depending if the first imaginary unit equals -1 or +1. You can find many more ways of crafting a multiplication but the best math results are always found in the complex and circular version of the numbers in that particular dimension…

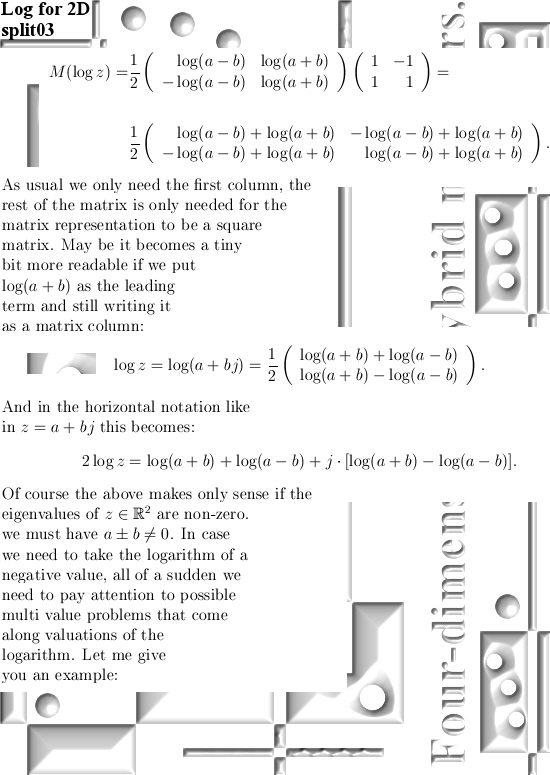
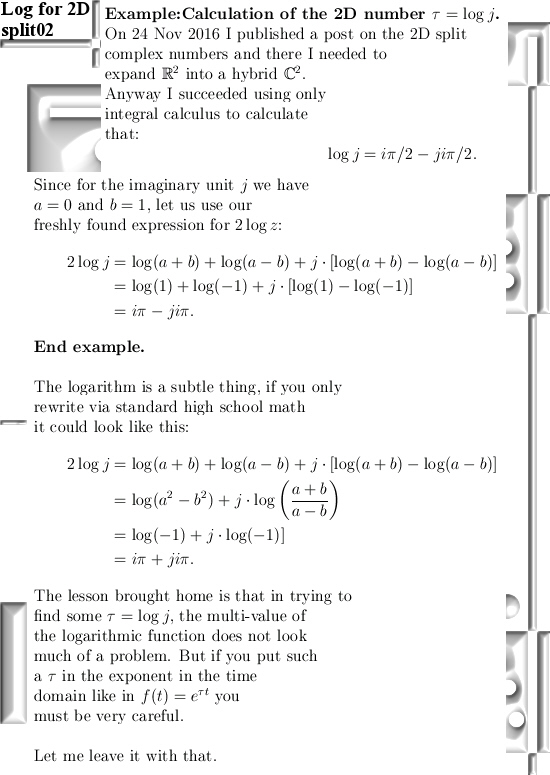
Ok, in this post I left all things out that talks about the 4D hybrid space that is a mixture of the 2D circular and complex numbers. But as you see on inspection of the above six pictures, the eigenvalues might be always real but they can be negative. As such always pay attention when you apply that function named the log…

That was it for this post, at this point in time I have no idea what the next post will be about. After all we had this long rout of over 20 posts on the 4D complex numbers and I left a whole lot of other stuff out in that period. Stuff like 3D Gauss integers or a general definition for integration that works in all dimensions. Till updates my dear reader.