It is about time for a new post on 3D numbers, circular and complex. In this post I write the sphere-cone equation in a matrix notation so see the previous post on conjugates if you feel confused. The sphere-cone equation gives us two equations, as the name suggests these are a sphere and a cone and on the intersection we find the famous exponential circle.

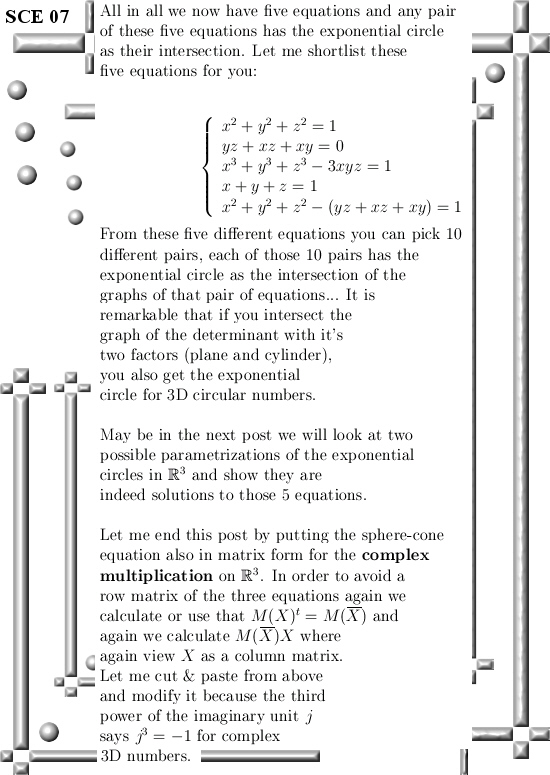
Beside the sphere-cone equation I also demand that the determinant equals 1, now we have three equations and every intersection of those 3 equations has as it’s solution the exponential circle. Can it become more crazy? Yes because it is possible to factorize the third degree determinant into a linear and a quadratic factor. Those factors must also be 1 and now we have five equations! And since you can pick 10 pairs out of five, we now have 10 ways of solving for the intersection where the exponential circle lives…

It is strange that after all these years it is still easy to find 10 video’s where so called ‘professional math professors’ sing their praise upon the exponential circle in the complex plane. They really go beserk over the fact that e to the power it gives the cosine and sine thing. And after all those years still silent, yeah yeah those hero’s really deserve the title of honorable shithole… It is honorable because they often have relatively large salaries and they are shitholes because of their brave behavior when it comes to 3D complex numbers. Bah, I am getting a bad taste in my mouth when I think about the behavior of professional math professors. Let me stop writing about that low form of life.

This post is 8 pictures long. May be, I have not decided yet, is the next post about parametrizations of the exponential circle. In these 8 pictures I work out the case for the circular multiplication, that is the case where the imaginary unit j behaves like j^3 = 1. At the end I only give the 3D complex version of the matrix form of the sphere-cone equation and the rest you are supposed to do yourself.

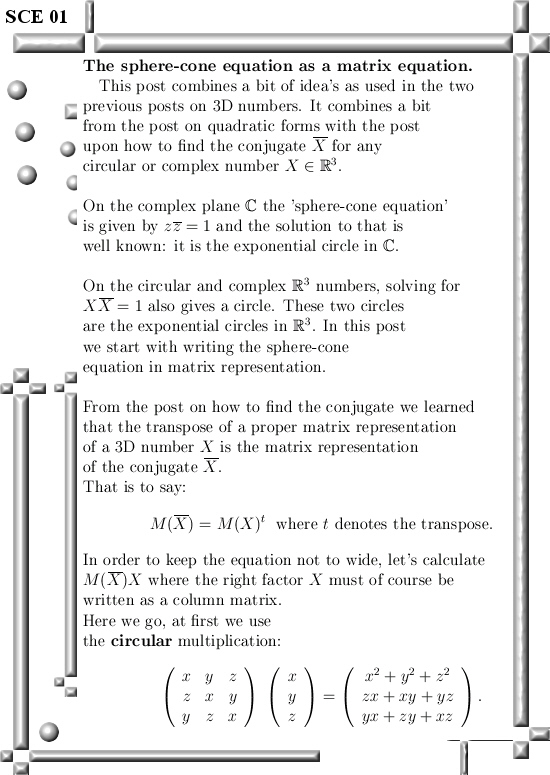
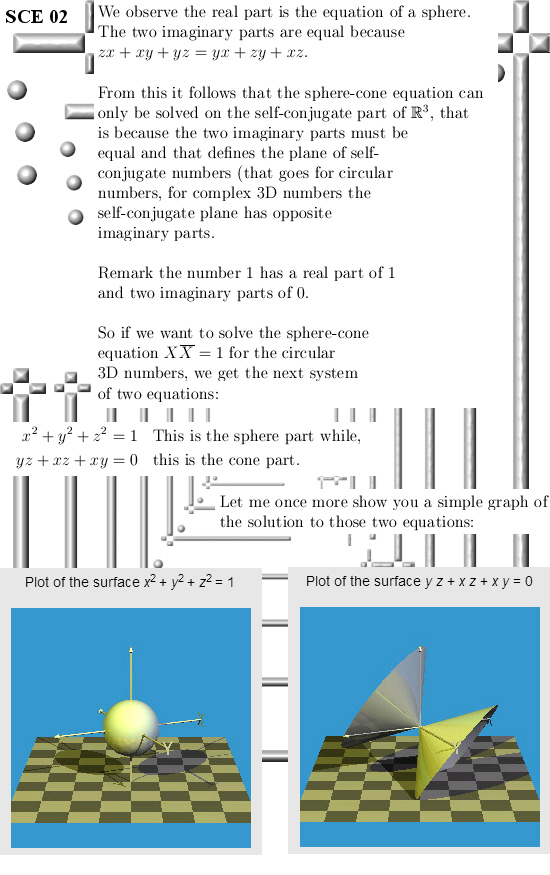
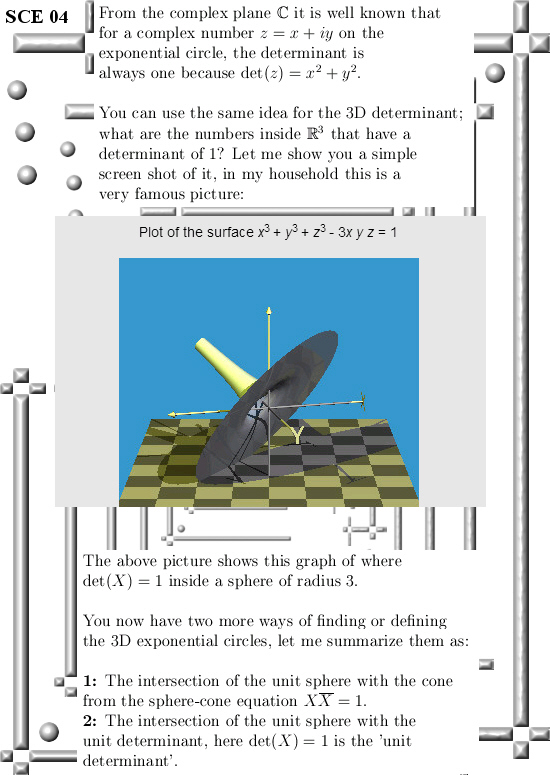
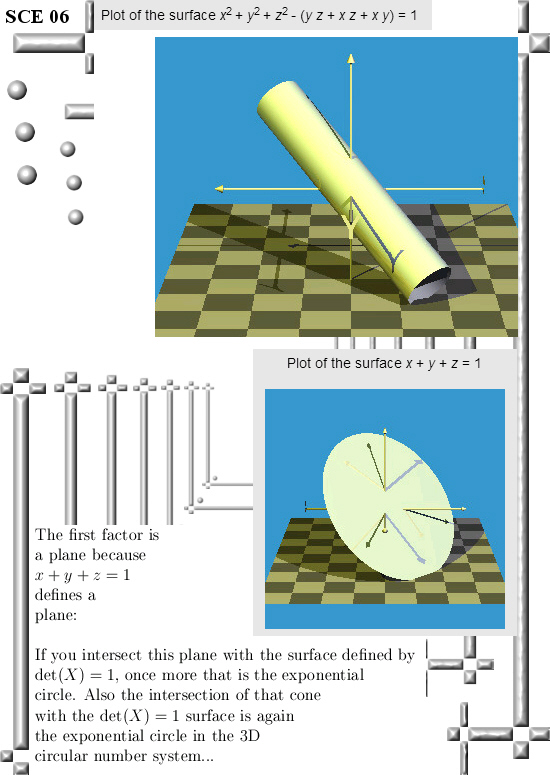
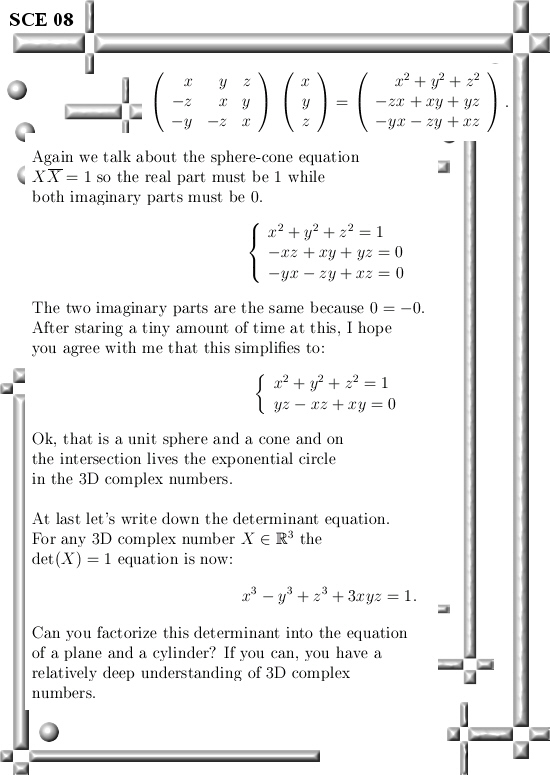
Ok, again do not confuse this with quadratic forms. A matrix equation as written above has a real and two imaginary components while quadratic forms are often just real valued.

Let´s try to upload this stuff. See you in the next post.