All in all it was a nice day today. Brewing is completed and tomorrow the wort can go into the fermentation bottles and the wonderful process of fermenting can take place. For those of you that also like to brew: A couple of months back I found a cute video explaining that you can also brew beer without cooking it. And I was like seeing water burning or I was like a professional math professor understanding 3D complex numbers for the very first time in their life… Anyway if you are interested search for ‘Raw ale no boil brewing’ on Youtube. It is of interest because if you brew without boiling, only after that you understand what you usually cook away in things that might taste good (or bad).
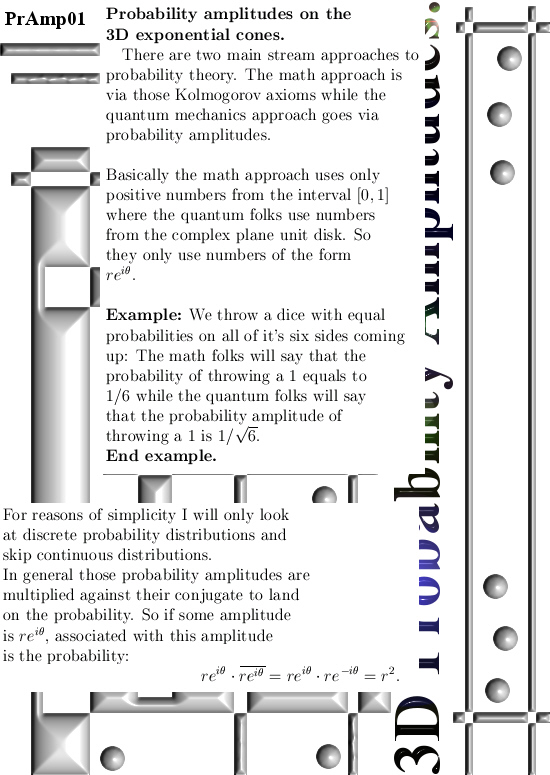
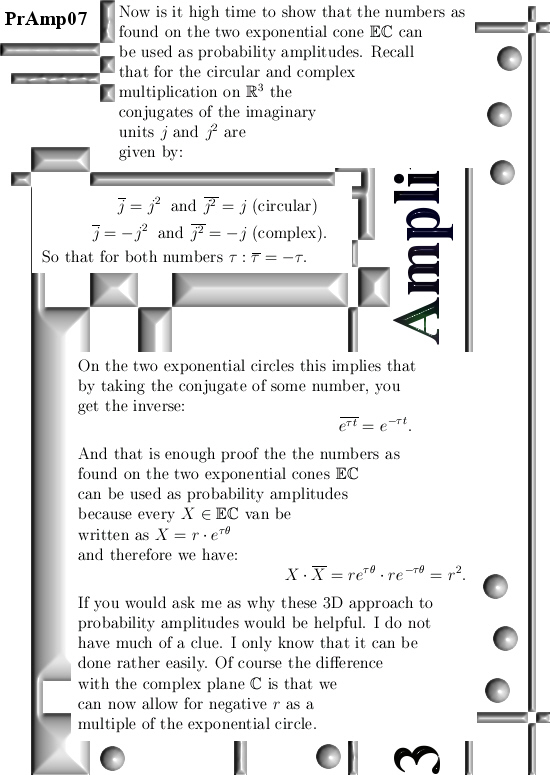
But let’s go to this post: It is about probability amplitudes as they are used in quantum physics where all those kind of amplitudes are multiplied against their conjugate and that gives a real positive number known as the probability. If you write it in polar coordinates on the complex plane, it is easy to see that those probability amplitudes can have all kinds of phases (the argument of the complex plane number). So for that to work on 3D complex or circular numbers, it would be great if you can write it more or less like the polar coordinates as in the complex plane. And that is easy to do in 3D space: Once you have found and also understand the exponential circles, it is evident that all numbers on those exponential cones are some real multiple of a number from the exponential circle.
As such the numbers found on the exponential cone can be written just like the polar stuff from the complex plane, also now the r as used in polar coordinates can also be negative. That is a strange result because for millions of years we were always indoctrinated by a positive r … 😉
Another important difference with the complex plane lies in the fact that the complex plane is closed under addition. That is obvious, but it is also obvious that on a cone it is very different. Most of the time if you add up two numbers you are either inside or outside the cone. But probability amplitudes are always multiplied against their conjugate and added up only later, so we can still use the exponential cone for things like that. I don’t see that ship stranding, so let’s do it.
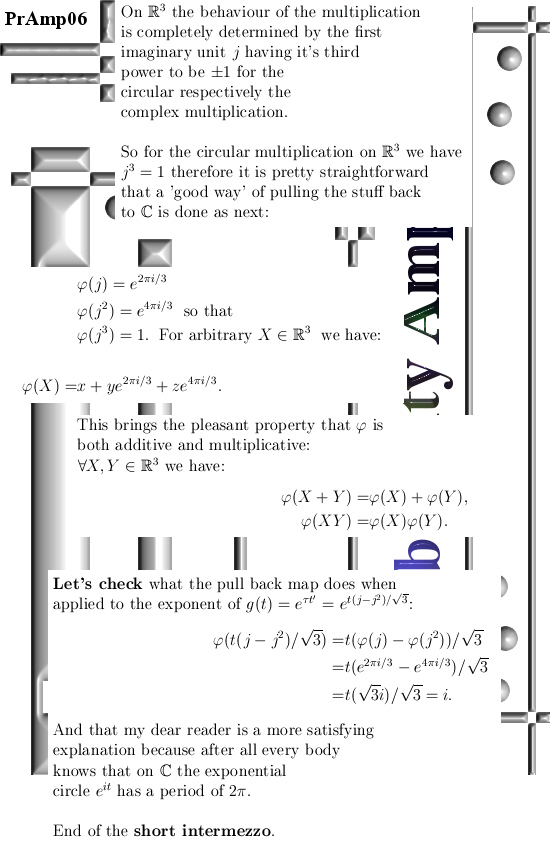
I also want to remark I am using the so called ‘pull back map’ once more. The professional professors also have a pull back map but that is a very different thing compared to what I use. So don’t be confused by that: the way I use it is to fix higher dimensional exponential circles (and curves) on the exponential circle in the complex plane. (This for fine tuning the period in time and stuff like that, or for understanding why the numbers are what they are: WTF that square root of 3 in it???
This post is 7 pictures long, most are the usual size of 550×775 pixels. At last I want to remark that for myself speaking I do not know if there is any benefit in trying this kind of use of 3D complex and circular numbers. It is funny to think about positive and negative values for r like for example in electron spin or a wave function for the electron pair. But I just do not know if this add any value or that you can use the complex plane only and miss nothing of all you could have learned.
Ok, here we go:
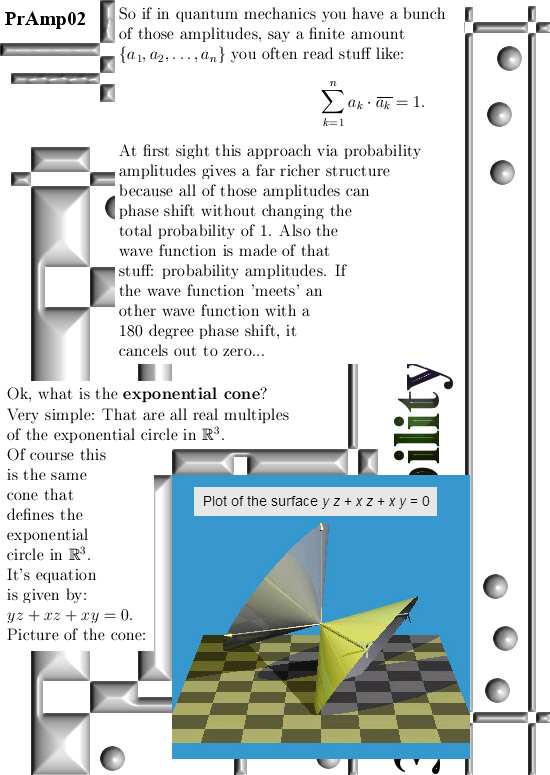
Ok, that was it for this post. Till updates my dear reader.