Monthly Archives: April 2020

Three video’s for killing the time if needed.

Exponential circle, Magnetism

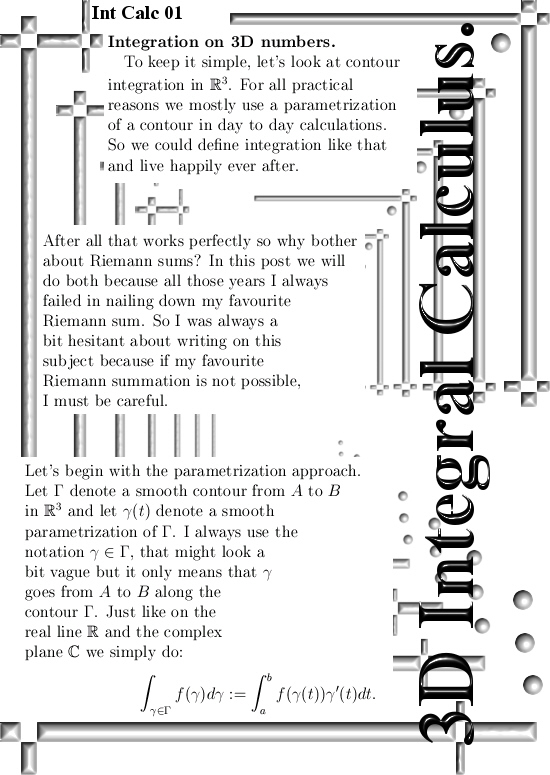
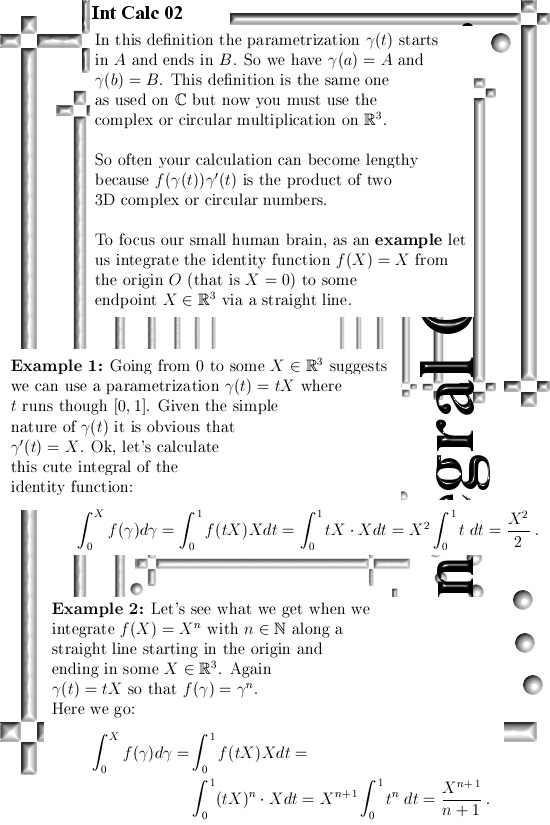
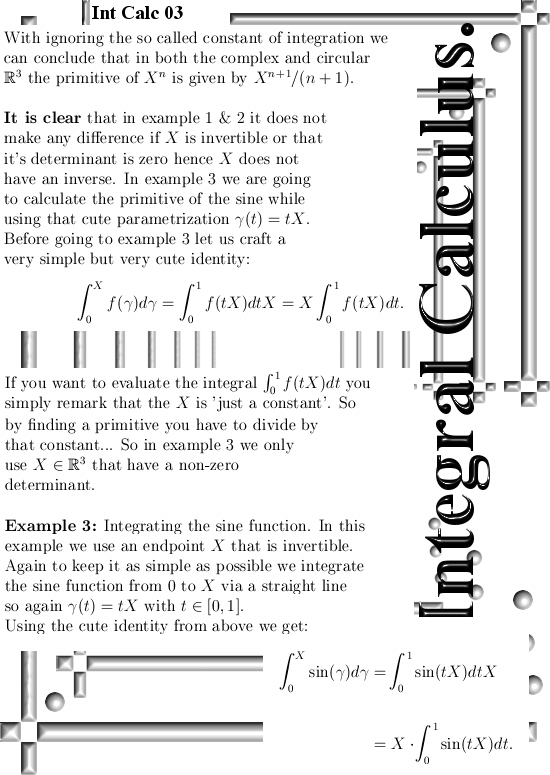
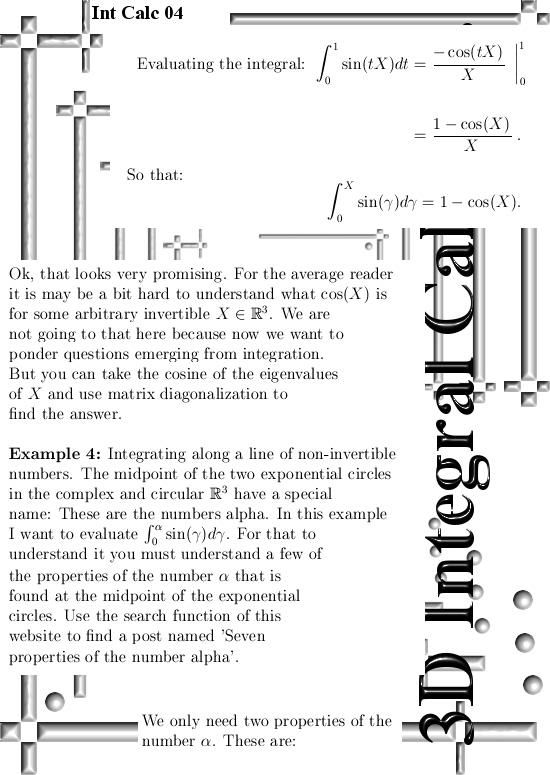
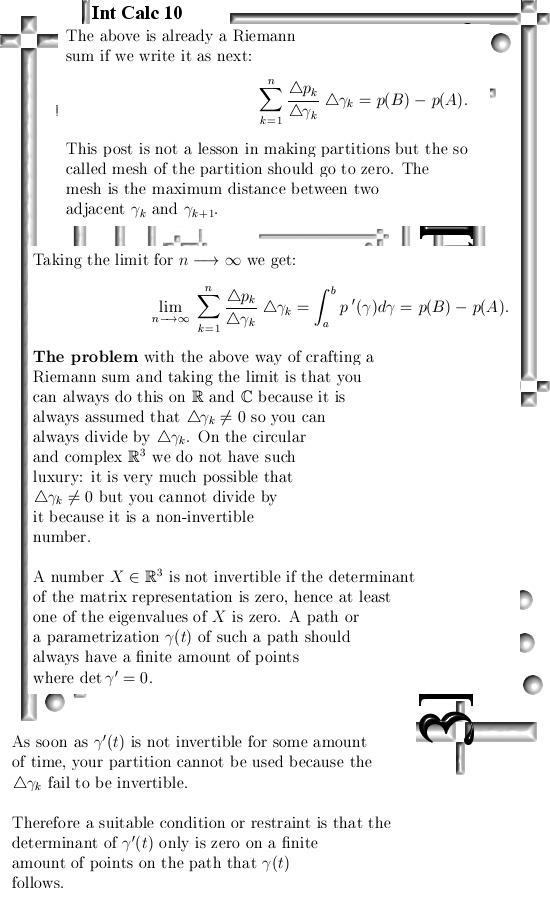
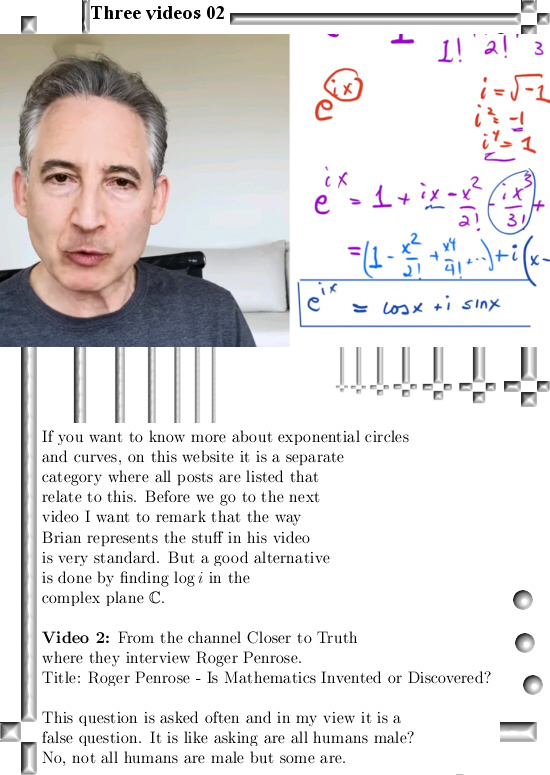
This time a somewhat different post, just 3 video’s I thought are interesting to share for their own reasons. In the first video the American television physics professor Brian Greene goes beserk on the beauty of the exponential circle in the complex plane… Brian, like so many others, do not know what they are missing. So many spaces have exponential circles and curves and indeed they are beautiful.

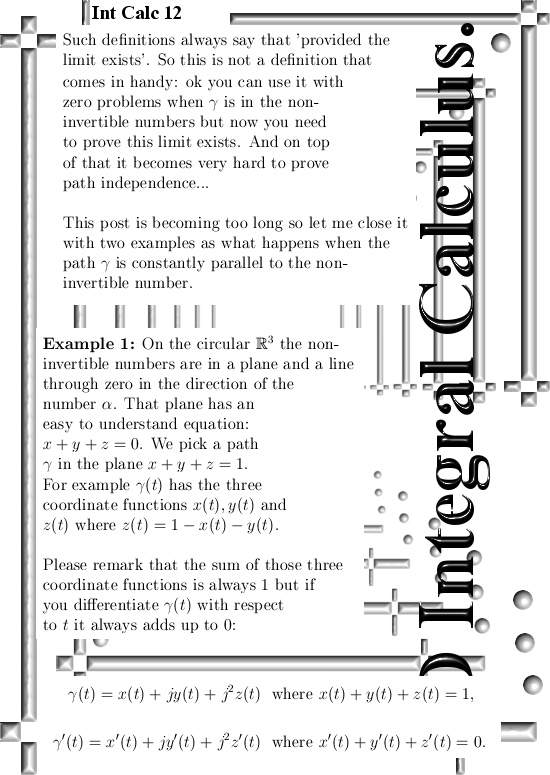
The second video is about a question that is often asked: Is math invented or is it a discovery? I think this is a false way of looking at math, if you replace the word ‘math’ by ‘food’ you already understand this is a weird question: Is food invented or is it discovered? In my view that often goes hand in hand but opinions vary wildly on this subject. The video is an interview with the UK math professor Roger Penrose. I included this video because back in the 80-ties of the previous century Roger had written some books on the things known as spinors. A lot of so called scientists think that spinors have something to do with electron spin, there are even weirdo’s that think after the electron has encircled the nucleus once it’s spin state is altered so that after two rounds the electron has it’s original spin back… Oh oh for people like Roger and those others it will be a long way in understanding the electron cannot be a magnetic dipole. In all ways possible that is not logical. For example the unpaired electron is not magnetically neutral while the electron pair is. And there are a whole lot more examples to be given showing electrons simply can’t be magnetic dipoles. And you only have to use the thing called logic for that; no weird quantum mechanical stuff but just a magnetic charge on the electron gives much better results if you use the thing called logic.

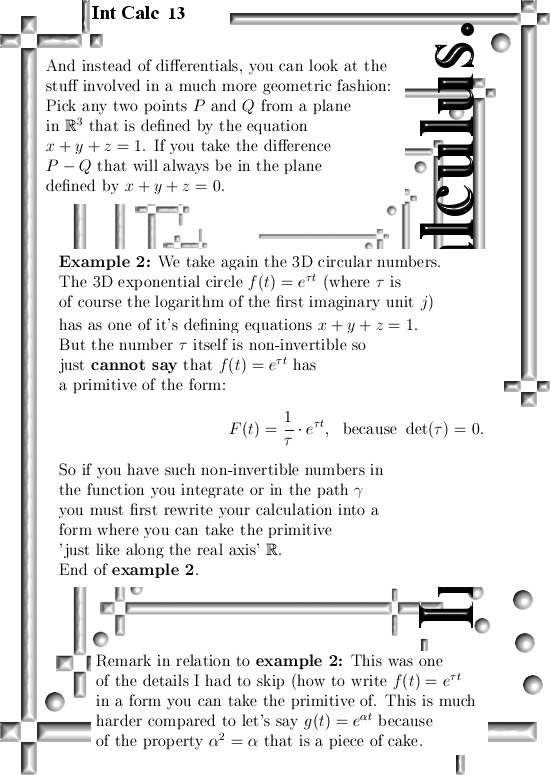
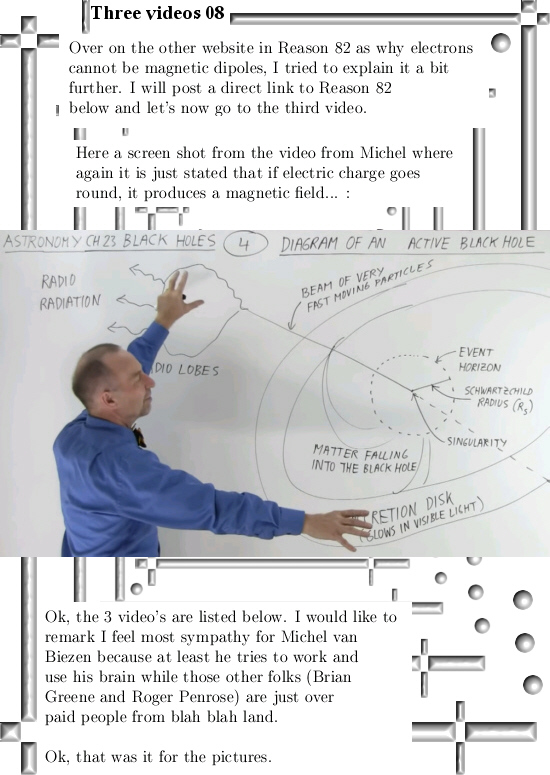
The third video is about a weird line of reasoning that I have observed in many video’s. It is about explaining how those jets form that emerge from black holes and their accredion disks. The reasoning is that the plasma in the accretion disk goes around the black hole and if a charge goes round it produces a magnetic field & that is all explanation given always. That is nonsense of course, even spinning metals like when you are drilling a hole with your drill machine never produces a magnetic field because for every electron that goes round on average also a proton goes round and all in all there is no overall magnetic field created. But if the electrons are magnetic monopoles, they will have much more acceleration compared to the far more heavy protons and as such an accretion disk around a black hole should be positively charged all of the time and that explains why the magnetic fields are so strong over there.

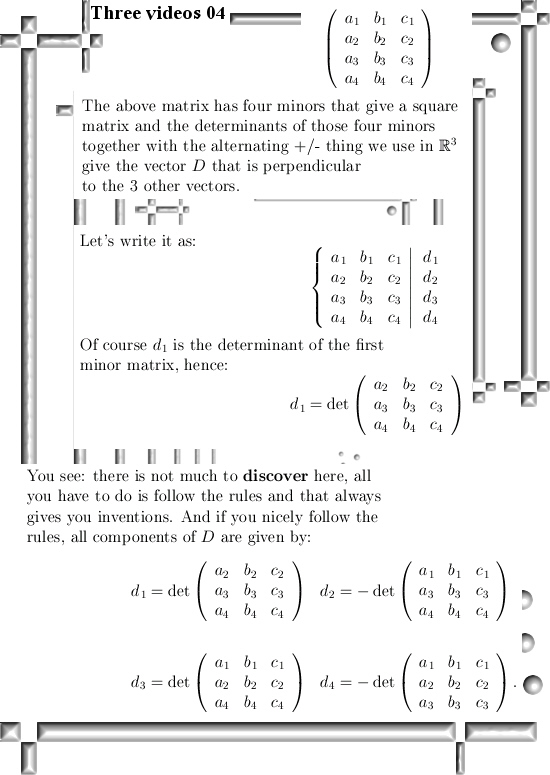
Ok, I crafted 8 pictures from the stuff. For example I made a 4D generalization of the 3D outer product while explaining such math is an invention and not a discovery. After the 8 pictures I will post the three video’s that aroused my attention for one reason or another. Have fun reading it.

The link to Reason 82 as why electrons cannot be magnetic dipoles is
08 Feb 2020: Reason 82: More on solar flares.
http://kinkytshirts.nl/rootdirectory/just_some_math/monopole_magnetic_stuff05.htm#08Feb2020

And here are the three Youtubers to kill the time.

Ok, let´s try to upload this bunch of stuff and see what happens.

Integration on the complex and circular 3D number spaces.

3D complex numbers, Integration

A lot of math professionals rather likely still think that 3D complex numbers do not exist, may be for reasons like there are non-invertible numbers or whatever what other reason they have. This post more or less proved such views are nonsense; for example a lot of math on the 2D complex plane does not rely on the fact it is a field (and as such only division by zero is forbidden).

But on the 3D complex and circular number spaces indeed it brings some complications if you have non-invertible numbers in the function you want to integrate over a particular curve. And I have to say that problem could be solved by using the special properties that those numbers have. In this post I only show some examples with the non-invertible number alpha (alpha is the midpoint of the 3D exponential circles and all multiples of alpha are also non-invertible so the line through 0 and alpha are all not invertible).

For me writing this was a good distraction away from all that negative news we have day in day, all those countries reporting daily death toll can make you a bit depressed… So when I am through with the daily news I always do some other stuff like calculating a few of such integrals. That is a very good antidote against all that bad news. After all there is not much gained if you constantly think about things you cannot change at all.

This post is relatively long; at first I crafted 12 pictures but it soon turned out that was not enough. So while filling the 12 pictures with the math and the text I expanded some of the pictures so they could contain more math & text. That was not enough and in the end I had to craft two more background pictures. All in all it is 14 pictures long, that is a record length for this website.

If in your own mathematical life you have performed contour integration in the complex plane, you must be able to understand how this works in the 3D spaces. And for those who have done the thing known as u-substitution on the real line: it is just like that but now this u thing is the parametrization of a path. All that stuff below with gamma in it is either the path or the parametrization of that path. Please remark that you must use the complex or the circular multiplication on 3D, just like integrating over a contour in the complex plane uses the 2D complex multiplication.

In case if you are not familiar with the number alpha that is found at the center of the exponential circle, use the search function of this website and for example look up ‘seven properties of the number alpha’.

I hope I have removed all faults, typo’s etc so that later I do not have to repair the math because that is always cumbersome. Here we go: 14 pictures long so this is hard to grasp in detail in just a few hours. But it is beautiful math & that is why I do this. For me math is a lovely hobby.

Enough of the blah blah blah, here we go:

Ok, let´s first hit the button ´Publish´ and see what will happen…
It looks all right but a day after first publication I realized there was some missing text. It turned out I had to rename picture number 2 and now every thing was like it was planned.

Later I will flea through the rest of the text, if needed I will post more addenda. For the time being that was it so till addendums or till the next post.