Last week I started thinking a bit about that second example from the pdf of Charles Frohman; the part where he projected a parallelogram on the three coordinate planes. And he gave a short calculation or proof that the sum of squares of the three projected areas is the square of the area of the original object.

In my own pdf I did a similar calculation in three dimensional space but that was with a pyramid or a simplex if you want. You can view that as three projections too although at the time I just calculated the areas and direved that 3D version of Pythagoras.

Within the hour I had a proof that was so amazingly simple that at first I laid it away to wait for another day or for the box for old paper to be recycled. But later I realized you can do this simple proof in all dimensions so although utterly simple it has absolutely some value.
The biggest disadvantage of proving more general versions of the theorem of Pythagoras and say use things like a simplex is that it soon becomes rather technical. And that makes it hard to read, those math formula’s become long can complex and it becomes harder to write it out in a transparant manner. After all you need the technicalities of your math object (say a simplex or a parallelogram) in order to show something is true for that mathematical object or shape.

The very simple proof just skips that all: It works for all shapes as long as they are flat. So it does not matter if in three dimensional real space you do these projections for a triangle, a square, a circle, a circle with an elleptical hole in it and so on and so on. So to focus the mind you can think of 3D space with some plane in it and on that plane is some kind of shape with a finite two dimensional area. If you project that on the three coordinate planes, that is the xy-plane, the yz and xz-plane, it has that Pythagoras kind of relation between the four areas.

I only wrote down the 3D version but you can do this in all dimensions. The only thing you must take in account is that you make your projections along just one coordinate axis. So in the seven dimensional real space you will have 7 of these projections that each are 6 dimensional…

This post is four pictures long, I did not include a picture explaining what those angles alpha and theta are inside one rectangular triangle. Shame on me for being lazy. Have fun reading it.

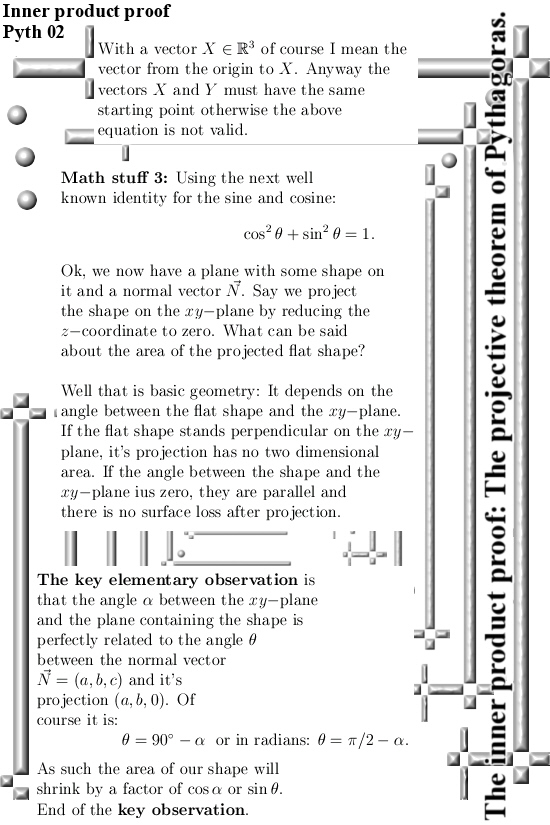
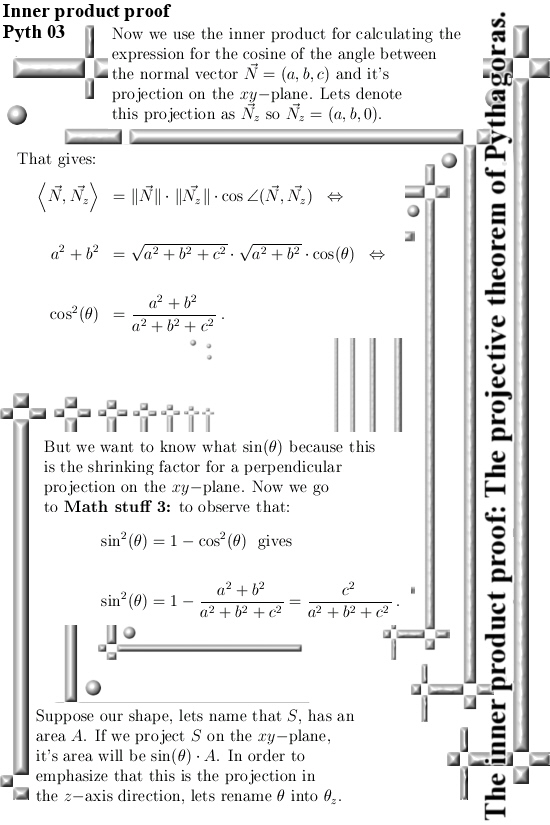
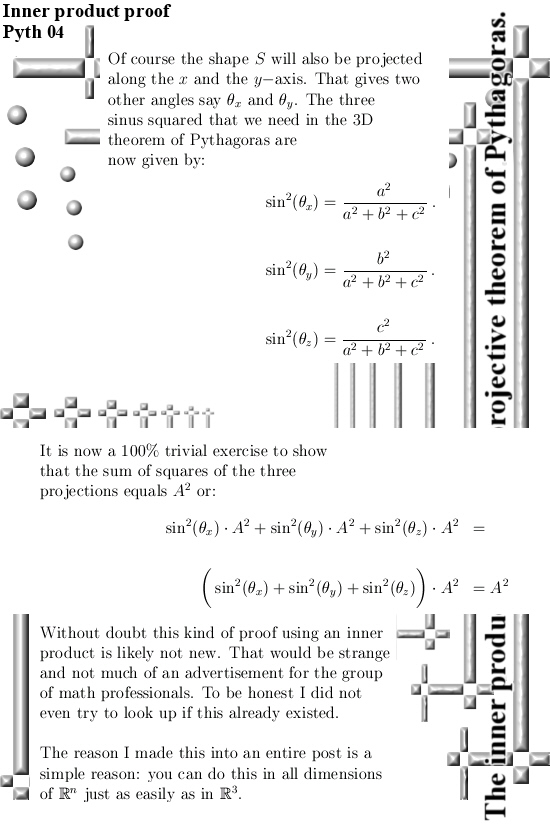
So all in all we can conclude the next: You can have any shape with a finite area and as long as it is flat it fits in a plane. And if that plane gets projeted on the three coordinate planes, the projected shapes will always obey the three dimensional theorem of Pythagoras.

Ok, thanks for your attention and although this inner product kind of proof is utterly simple, it still has some cute value to it.