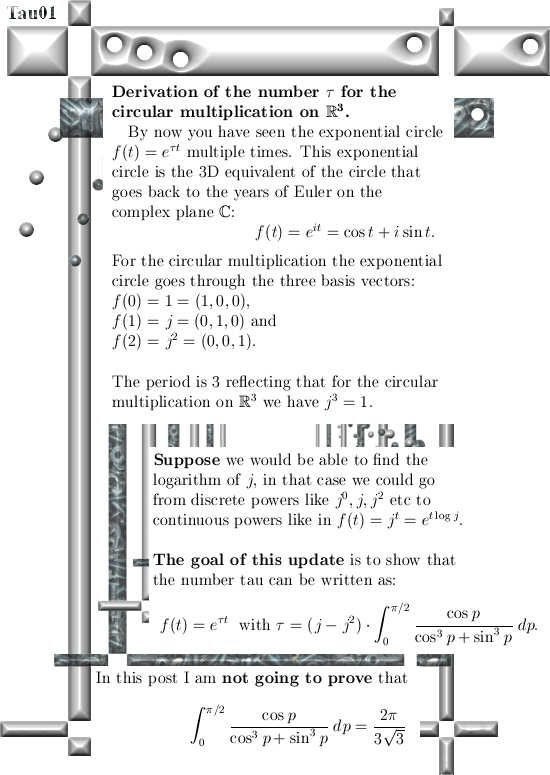
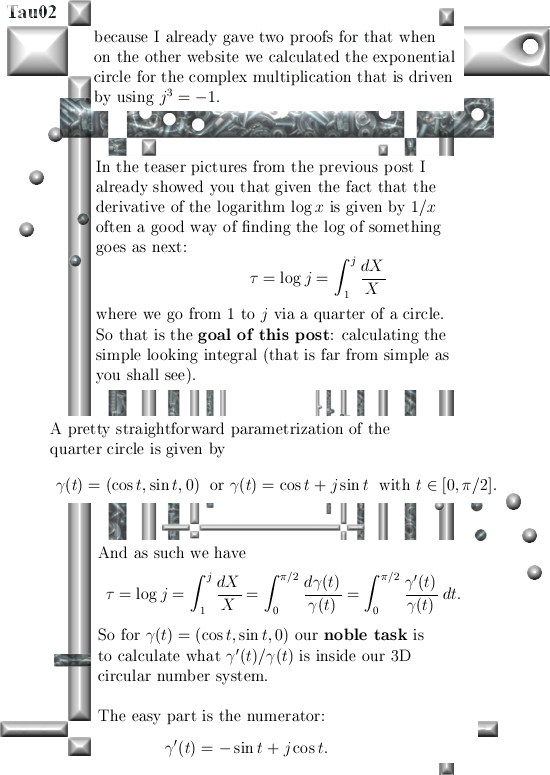
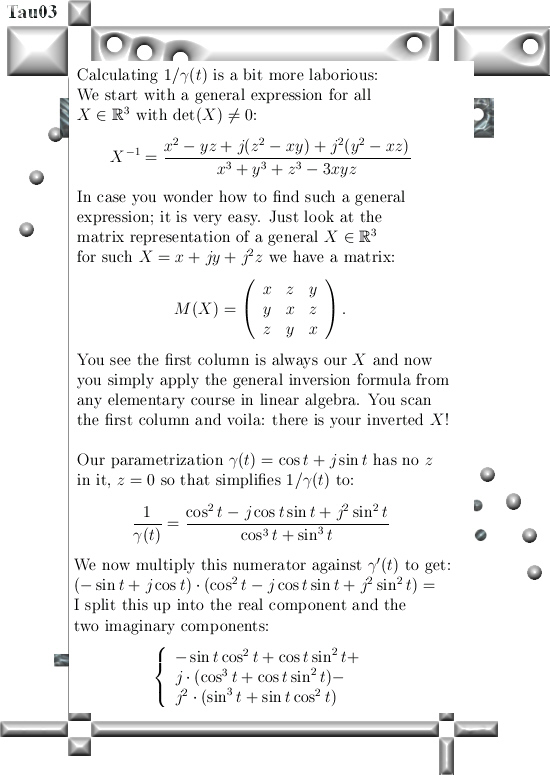
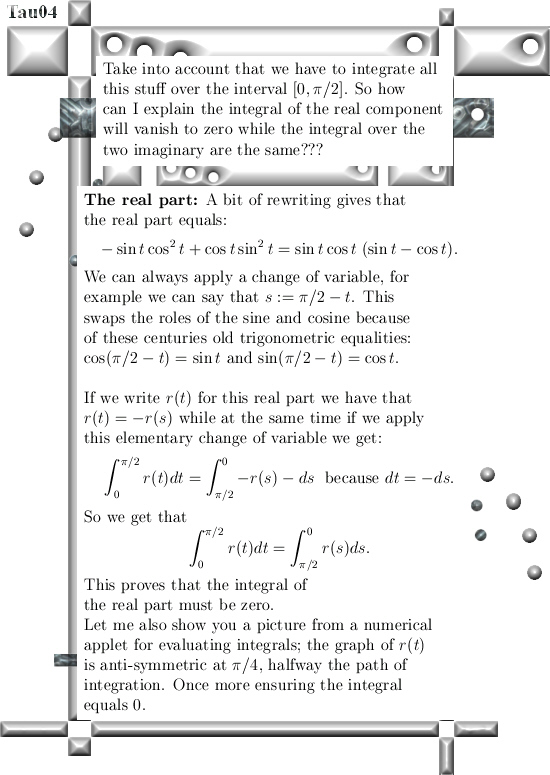
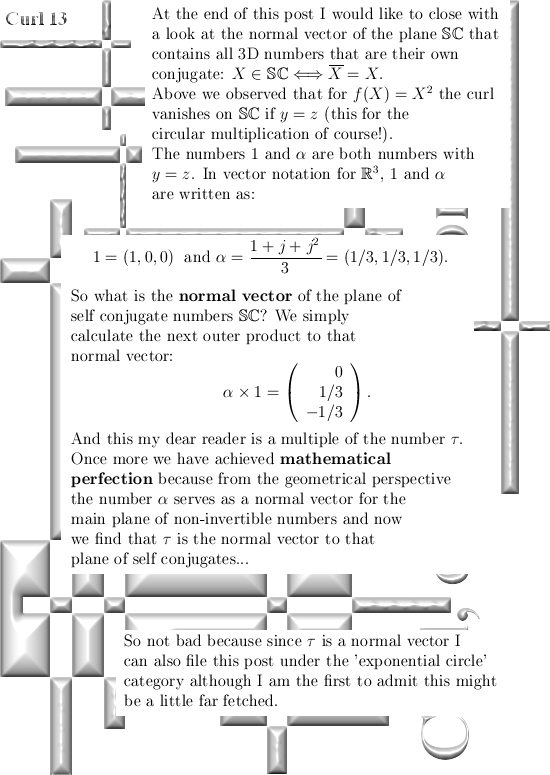
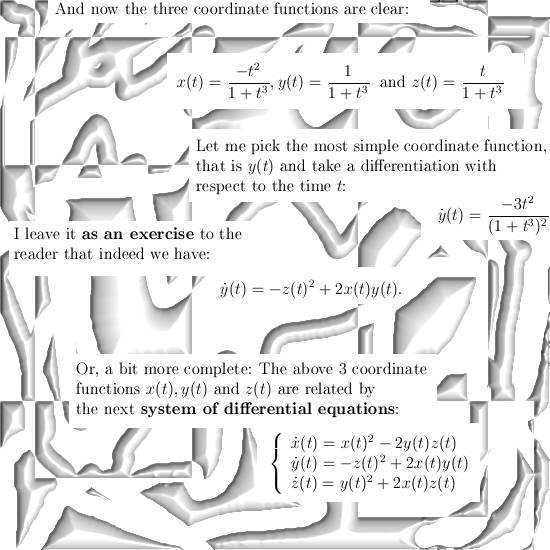
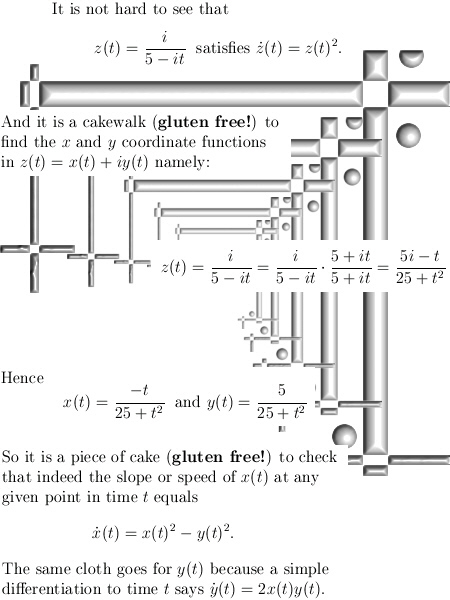
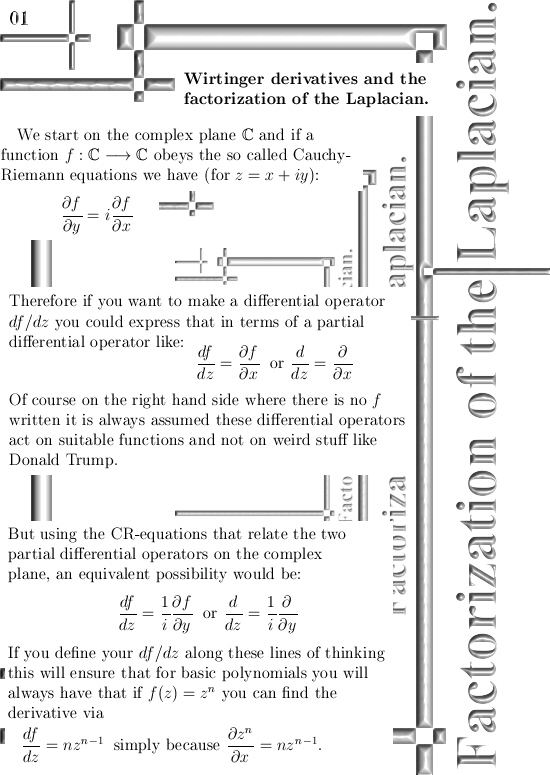
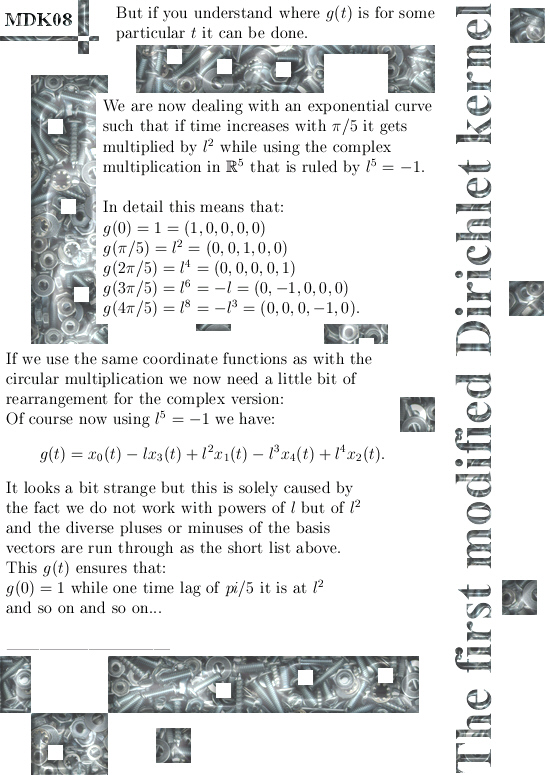
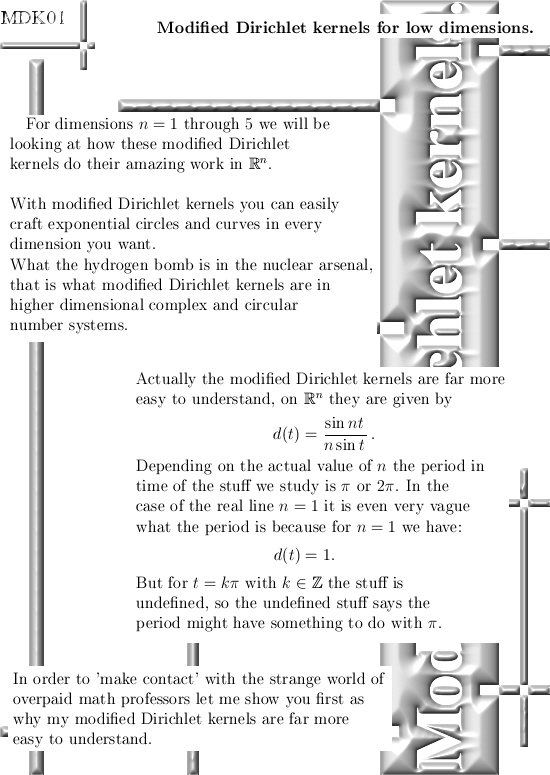
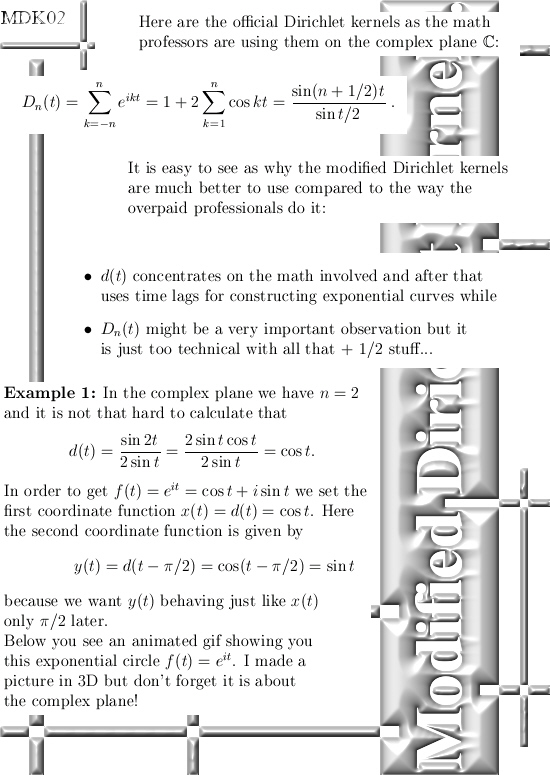
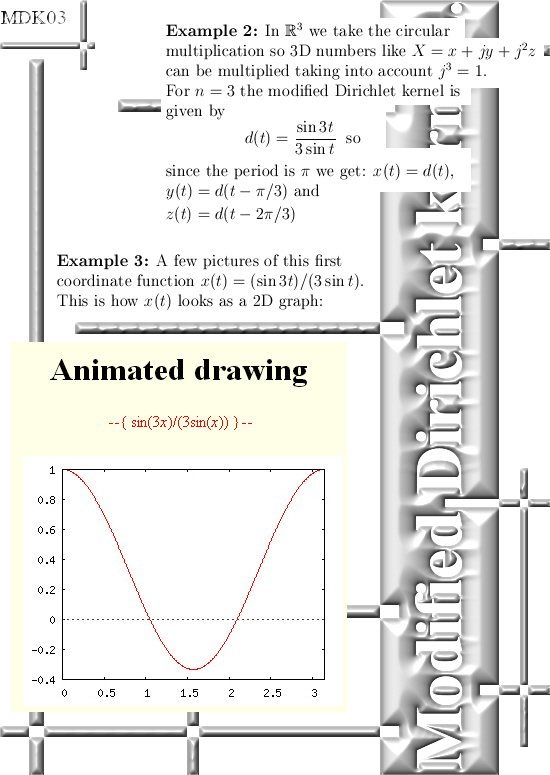
It has been a long time since my last update and that is caused by some stupid medical condition I still have and in my native language it is known as a ‘peesschede ontsteking’.
In practice this means I must do all typing on my computer keyboard with my left hand because in the evening I still cannot use my right hand.
Let me spare you the details but the long durance of the pain could even date back to the time when I was a dumb 15 year old with a broken wrist not seeking medical help.
So for the time being no long updates on perfect new hybrid number systems, it takes too much pain to write those long math stories down. So I retreat and just post a link to what is a very good Youtube video on the Riemann zeta function and it’s continuation into it’s analytic continuation.
Here is the video from the 3Blue1Browne guy:
Visualizing the Riemann zeta function and it’s analytic continuation
Nice vid isn’t it?
Last year on 26 March 2015 I wrote an update on where to find the zero’s of the Riemann zeta function in the 3D complex number system. I still consider this being an important publication although that human garbage known as the ‘professional math professors‘ said nothing all these months, I still think it is worth the trouble and try to post a new link to it:
From 26 March 2015: Zeta on the critical strip (3D version only).
http://kinkytshirts.nl/rootdirectory/just_some_math/3d_complex_stuff03.htm#26March2015
May be it is best to leave this update with that;
Zero point zero point zero point zero reaction of so called ‘professional math professors’ upon finding the zero’s of the Riemann zeta function in dimensions above 2.
Once an overpaid imbecile, always an overpaid imbecile.
Let’s leave it with that.
__________
Update from 19 Dec: I did not include yesterday a more easy to understand analytic continuation that I wrote myself this year; it is the analytic continuation of the geometric series and as such I am debunking the stuff some of the children of a lesser God seem to think:
1 + 2 + 4 + 8 + 16 + ….. = -1.
Nottingham professors from math and physics seem to think that
1 + 2 + 3 + 4 + 5 + ….. = -1/12.
This is also nonsense and there are many ways to prove this is not the case but inside theoretical physics this is actually used: that is the process of renormalization. Every time professional physics professors encounter an infinity in their calculations it is not that they say ‘Something must be wrong with our theory’. No if they encounter stuff like 1 + 2 + 3 + 4 + etc, they replace it by -1/12.
It works pretty well in order to get rid of those singularities they say.
Anyway here is the link to what I had to say on that subject:
From 15 April 2016: Debunking the Euler evaluation of zeta at minus one.
http://kinkytshirts.nl/rootdirectory/just_some_math/3d_complex_stuff04.htm#15April2016
You can find the analytic continuation of the geometric series in the fifth picture.
Let me close this extra update with the Youtube video from those weird weird Nottingham professors that started it all:
ASTOUNDING: 1 + 2 + 3 + 4 + … = -1/12
And indeed if it were true it would be very very astounding.
What for me is TRULY ASTOUNDING is that the very professors you see doing their show is that they think the harmonic series is divergent. The harmonic series is also the zeta function evaluated at 1:
1 + 1/2 + 1/3 + 1/4 + … = infinity.
So the Nottingham professors think that the harmonic series is divergent (that is correct of course) while the sum of all integers is convergent to be -1/12.
Welcome to the world of 21-th century science. Till updates.