In the previous post on 4D complex numbers I went a little bit philosophical with asking if these form of crafting a 4D number system is not some advanced way of fooling yourself because your new 4D thing is just a complex plane in disguise…
And I said let’s first craft the Cauchy-Riemann equations for the 4D complex numbers, that might bring a little bit more courage and making us a little bit less hesitant against accepting the 4D complex numbers.
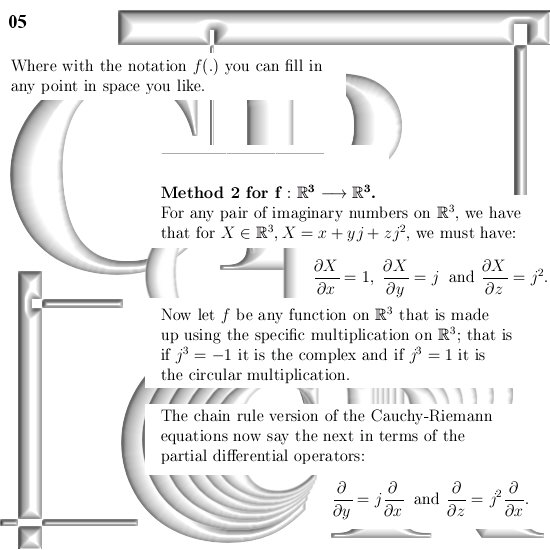
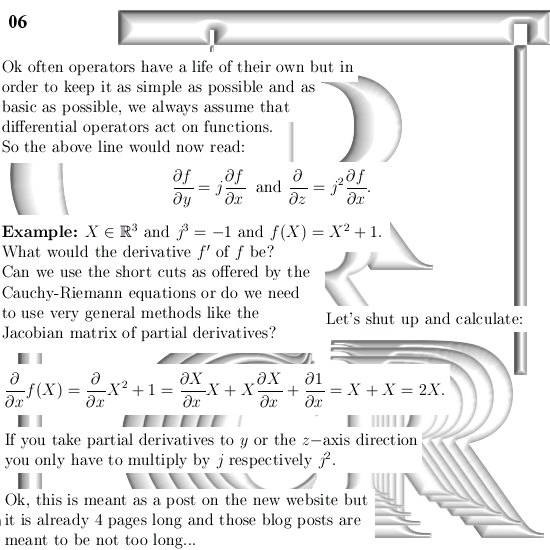
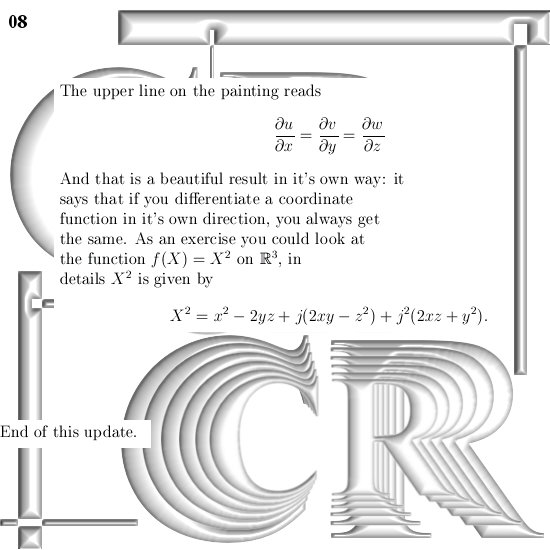
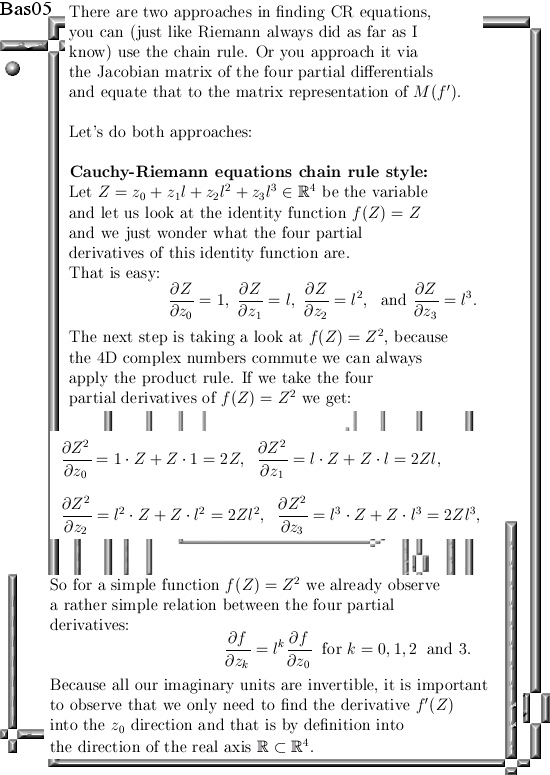
In this post we also do the CR equations and indeed they say that for functions like f(Z) = Z^2 you can find a derivative f'(Z) = 2Z. So from the viewpoint of differentiation and integration we are in a far better spot compared to the four dimensional quaternions from Hamilton. But the fact that the CR equations can be crafted is because the 4D complex numbers commute, that is XY = YX. And on the quaternions you cannot differentiate properly because they do not commute.
So crafting Cauchy-Riemann equations can be done, but it does not solve the problem of may be you are fooling yourself in a complicated manner. Therefore I also included the four coordinate functions of the exponential 4D curve that we looked at in the previous post.
All math loving folks are invited to find the four coordinate functions for themselves, in the next post we will go through all details. And once you understand the details that say the 4D exponential curve is just a product of two exponential circles as found inside our 4D complex numbers, that will convince you much much more about the existence of our freshly unearthed 4D complex numbers.
Of course the mathematical community will do once more in what they are best: ignore all things Reinko Venema related, look the other way, ask for more funding and so on and so on. In my life and life experiences not one university person has ever made a positive difference, all those people are only occupied with how important they are and that’s it. Being mathematical creative is not very high on the list of priorities over there, only conform to a relatively low standard of ‘common talk’ is acceptable behavior…
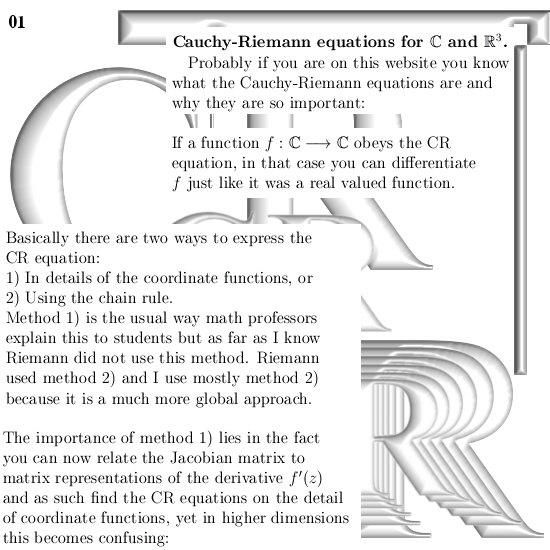
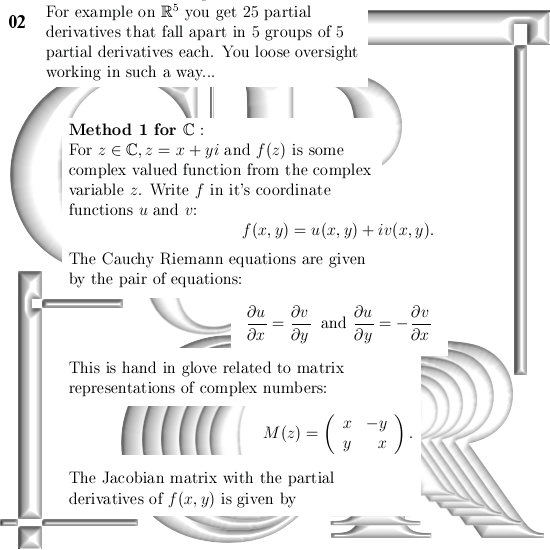
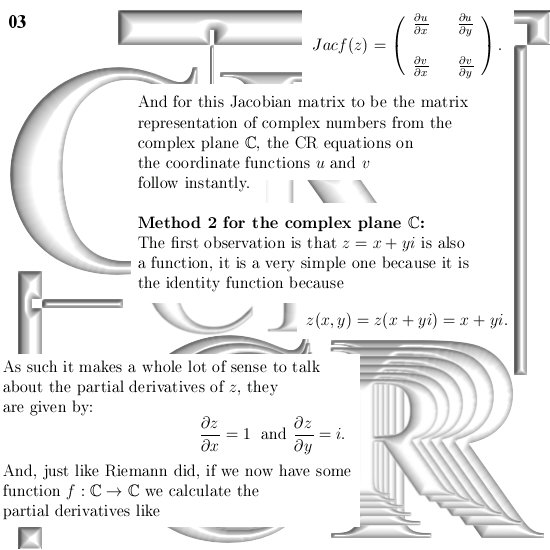
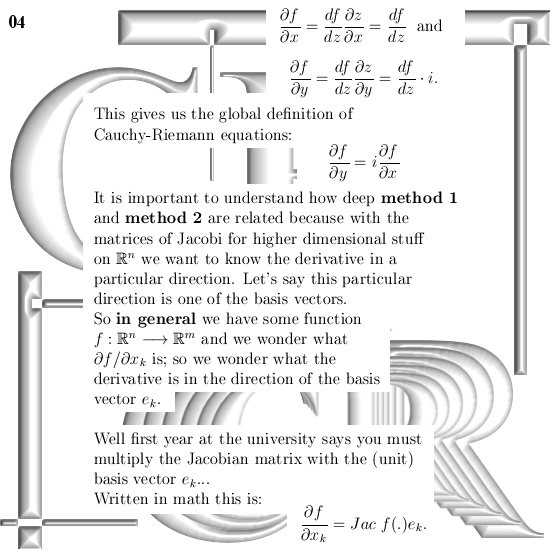
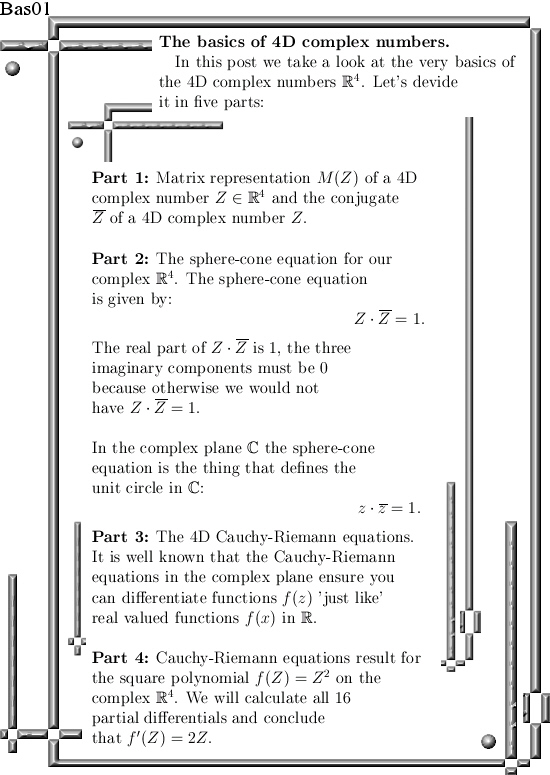
After having said that, this post is partitioned into five parts and is 10 pictures long. It is relatively basic and in case that for example you have never looked at matrix representations of complex numbers of any dimension, please give it a good thought.
Because in my file I also encountered a few of those professional math professors that were rather surprised by just how a 3 by 3 matrix looks for 3D complex numbers. How can you find that they asked, but it is fucking elementary linear algebra and sometimes I think these people do not understand what is in their own curriculum…
Ok, here are the 10 pictures covering the basic details of 4D complex numbers:

Ok, that was the math for this post.
And may be I am coming a bit too hard on the professional math professors. After all they must give lectures, they must attend meetings where all kinds of important stuff has to be discussed until everybody is exhausted, they must be available for students with the questions and problems they have, they must do this and must do that.
At the end of the day, or at the end of the working week, how much hours could they do in free thinking? Not that much I just guess…
Let’s leave it with that, see you in the next post.