Category Archives: Last Fermat theorem

The last Fermat theorem (positive version) versus the number 1.

Last Fermat theorem, Uncategorized

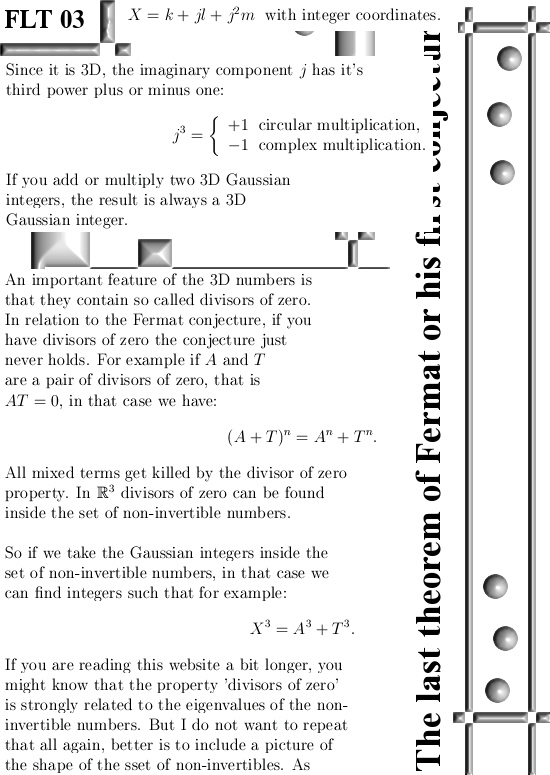
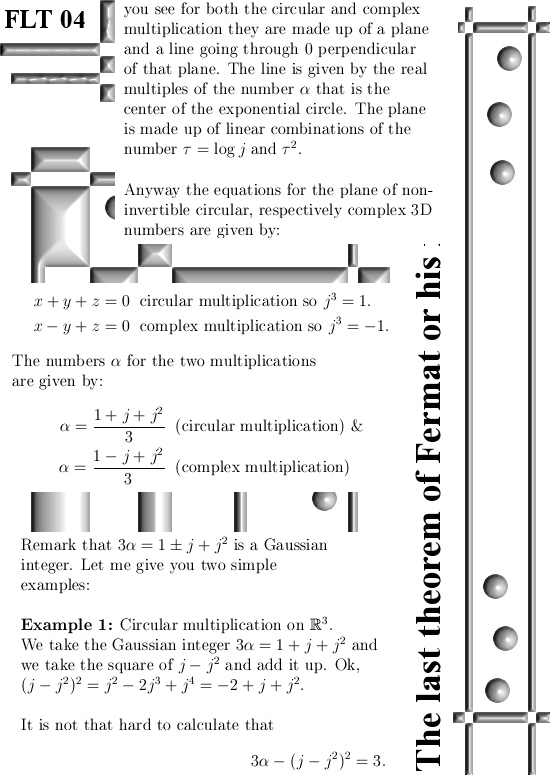
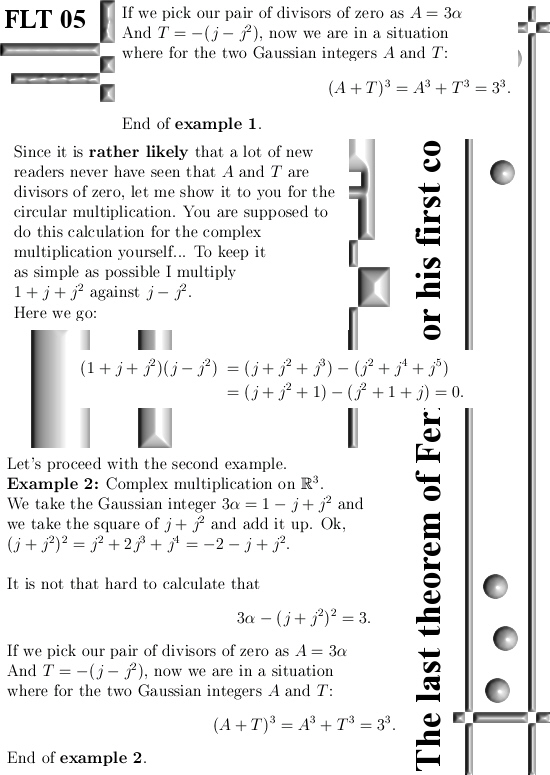
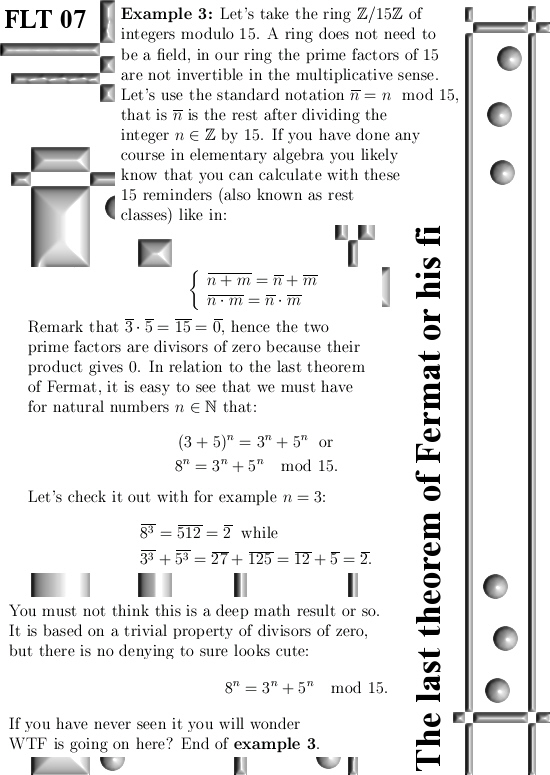
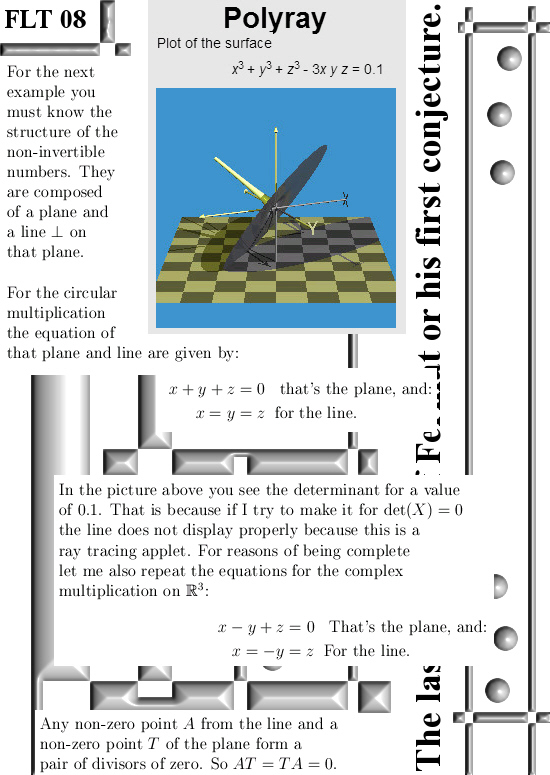
This is a short post; just over 3 pictures long. We make a few calculation on the ring of integers modulo 35. Of course that is a ring and not a field because 35 has two prime factors namely p = 5 and q = 7. These two prime factors form so called divisors of zero, that means that pq = 35 = 0 inside the ring of integers modulo 35.
Because the two prime factors have this property, that has all kinds of simplications when it comes to expanding (p + q)^n inside this ring. That is what I name the ‘positive version’ of the last theorem of Fermat: The ring of integers modulo 35 is a simple number space where the last theorem of Fermat is possible, here we again have 12^n = (5 + 7)^n = 5^n + 7^n.

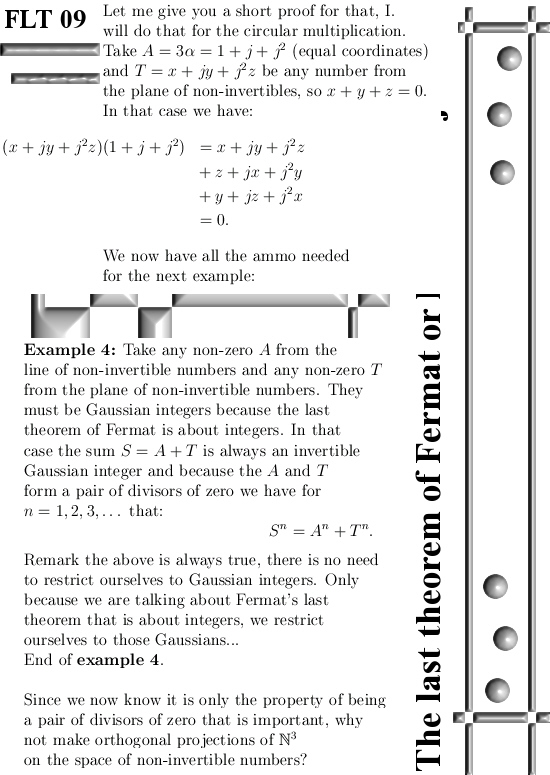
In this post I use the fact that the prime numbers 5 and 7 are also relatively prime and as such you can make a linear combination of them to get the number 1. And once you have the number 1 you can use them as a basis for the entire ring of integers modulo 35. But if you have a healthy brain, likely you will remark that it is far more easy to just use the counting numbers 1, …, 35 or just 1 to craft such a basis… So I understand that you might think I am crazy to the bone. Of course I am crazy to the bone but there is a goal in this utter madness. Take for example 3*5 – 2*7 = 1, this is one possibility to form the number 1 as a linear combination of 5 and 7. Since both terms contain one of the pairs of divisors of zero as a factor, this linear combination allows for a positive last theorem of Fermat decomposition: For a natural numbers n we have that: (15 – 14)^n = 15^n + (-1)^n*14^n = 1.
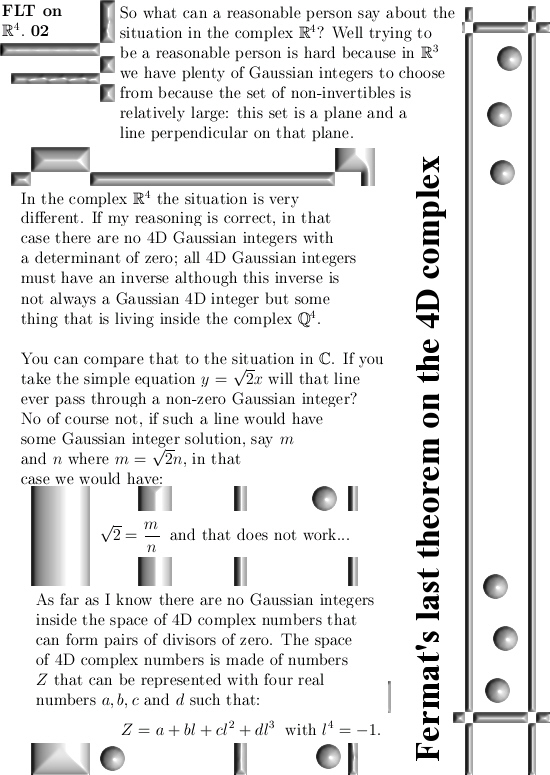
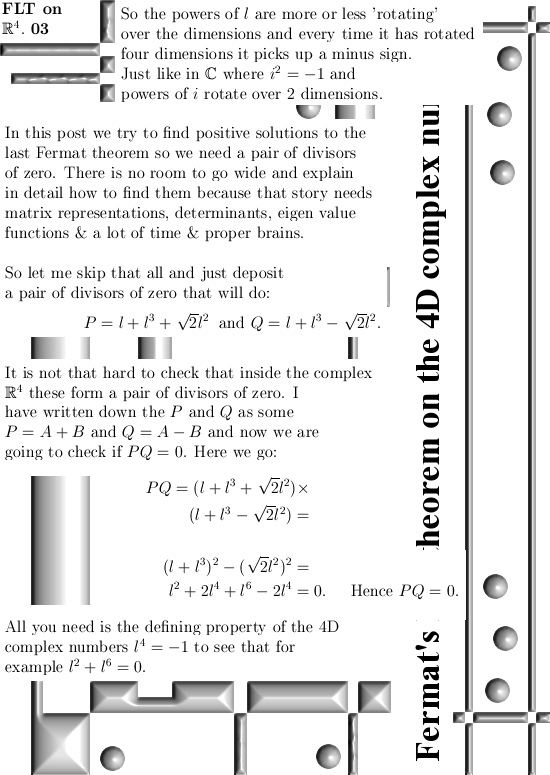
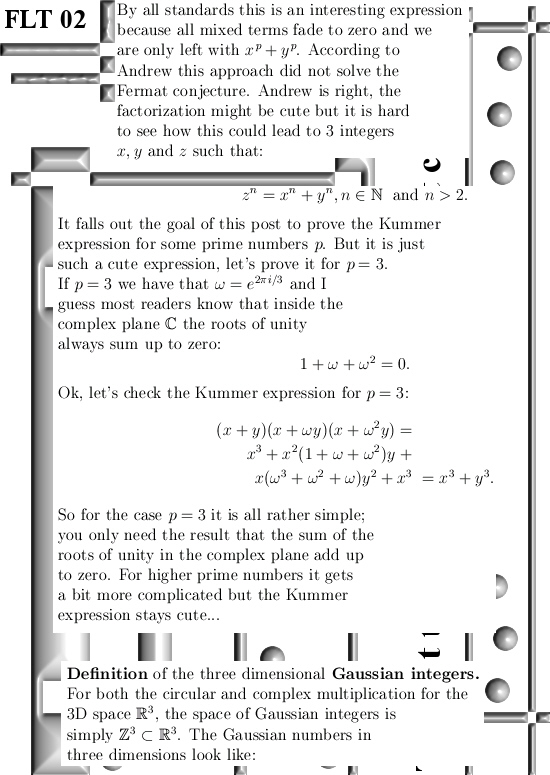
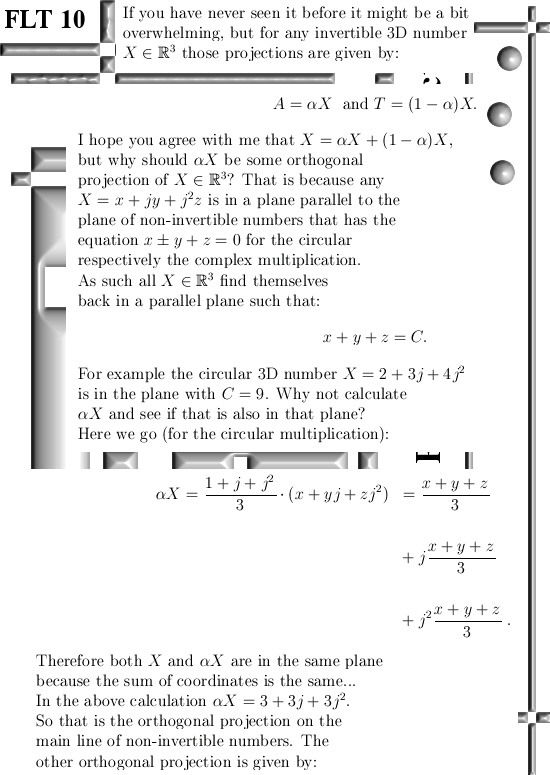
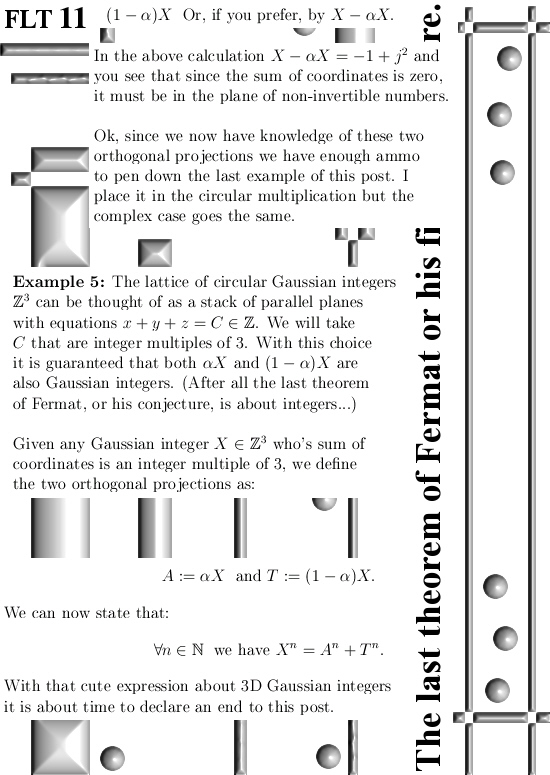
Although such expressions are very cute looking, it has no significant math depth anyway. All in all this post it totally unimportant because it is all so simple. The post upon the 3D Gaussian integers is far more important because there it was possible to write the number 3 as a linear combination of two 3D Gaussian integers. As such for the first time in about 350 years it was the first serious counter example against the last theorem of Fermat because that number 3 was just on the line of integers. It was not something inside some modulo number space or so, that was the real deal for the first time in 350 years.

Will math professors react on such a finding? Of course not. For example they would reason before the finding that if you can’t use 3D complex numbers to find only one significant result in algebra or number theory, that proves 3D complex numbers are useless.
And after the counter example to the last fermat theorem? Well math professors are the most smart people on earth, they are higly agile and adeptable and now the reasoning will likely be something like: In the entire history of mathematics nobody has ever used 3D Gaussian integers. This all is so far fetched that this is not serious math…

Well that is how they are and there is no changing that kind of behavior I just guess. Anyway enough of the blah blah blah. The post is just over 3 pictures long, has no mathematical significance anyway and I hope you have some fun reading it.

For odd n you get a minus sign, for even n you get a plus sign.
It is not significant math, but it sure looks very cute!

It is now one hour after mdinight so it is time to hit that button named ‘Publish website’. Live well & think well my dear reader. See you in the next post or so.

Another counter example to Fermat’s last theorem using 4D complex numbers.

4D complex numbers, Last Fermat theorem

All in all I am not super satisfied with this post because the math result is not that deep. Ok ok the 4D complex numbers also contain non-invertible numbers, say P and Q, and these are divisors of zero. That means PQ = 0 while both P and Q are non-zero. And just like we did in the case of 3D circular and complex numbers because of the simple property PQ = 0 all mixed terms in (P + Q)^n become 0 and as such: (P + Q)^n = P^n + Q^n.

In the space of 4D complex numbers an important feature of the determinant det(Z) of a 4D complex number Z is that it is non-negative. As such there is not a clear defined layer between the part of the number space where the determinant is positive versus the negative part. During the writing of this post it dawned on me that Gaussian integers in the 4D complex space always have a non-zero determinant. As such the inverse of such a Gaussian exists although often this is not a Gaussian integer just like the inverse of say the number 5 is not an integer. A completely unexpected finding is that the 4D complex fractions form a field…

That made me laugh because the professional math professors always rejected higher dimensional complex numbers because they are not a field. For some strange reason math professors always accept or embrace stuff that forms a field while they go bonkers & beserk when some set or group or ring is not a field. This is a strange behavior because the counter examples that I found against Fermat his last theorem are only there because 3D and 4D numbers are not a field: there are always non zero numbers that you cannot invert.
As such a lot of math professors are often busy to make so called field extensions of the rational numbers. And oh oh oh that is just soo important and our perfumed princes ride high on that kind of stuff. And now those nasty 4D complex numbers from those unemployed plebs form a field too…
I had to smile softly because 150 years have gone since the last 4D field was discovered, that is known as the quaternions, and now there is that 4D field of rationals that are embedded into something the cheap plebs name ‘4D complex numbers’? How shall the professional math professors react on this because it is at the root of their own behavior over decades & centuries of time?

Do not worry my dear reader: They will stay the overpaid perfumed princes as they are. Field or no field, perfumed princes are not known to act as adult people.

After having said that, this post is only five pictures long all of the ususal size of 550×775 pixels. For myself speaking I like the situation on the 3D numbers more because there you can easily craft an infinite amount of counter examples against the last theorem of Fermat.
Ok, here we go:

Yes I have to smile softly: all this hysteria from overpaid math professors about stuff being a field or not. And now we are likely into a situation where the 4D complex numbers are not a field but the space of 4D complex rationals is a field…

Will the math professors act as adults? Of course not.
Ok, let’s end this post because you just like me will always have other things to do in the short time that we have on this pale blue dot known as planet earth. Till updates.

The last theorem of Fermat does not hold for the 3D so called Gaussian integers.

3D complex numbers, Last Fermat theorem

On the one hand it is a pity I have to remove the previous post from the top position. Never ever I would have thought that the Voyager probes would be a big help in my quest of proving that electrons are not magnetic dipoles. Electrons are magnetic monopoles, if your local physics professor thinks otherwise why not ask you local physics professor for the experimental evidence there is for the electron magnetism dipole stuff?

On the other hand this post is about Gaussian intergers for the 3D complex and circular numbers and it is with a bit of pride that I can say we have a bunch of beautiful results because the last theorem of Fermat does not hold in these spaces.

The last theorem of Fermat is a kind of negative result, it says that it is impossible for three integers x, y and z that x^n + y^n = z^n, this for integer values of n greater than 2 of course. (For n = 2 I think most readers know it is possible because those are the Pythagoras triples.)

Anyway I succeeded into writing the number 3 as the sum of two Gaussian 3D integers that are also divisors of zero. So this pair of integers, in this post I name them A and T because they are related to the famous 3D numbers alpha and tau, are divisors of zero so as such AT = 0. As such as a denial of the Fermat theorem, an important result as posted here is that A^n + T^n = 3^n. So on the 3D complex & circular numbers this result is possible while if you use only the 2D complex plane and the real line this is not possible…
But there are plenty of spaces where the Fermat conjecture or the last theorem does not hold. A very easy to understand space is the ring of integers modulo 15. In this ring there are numbers that do not have a multiplicative inverse, say 3 and 5. And if inside this ring you multiply 3 and 5 you get 15 and 15 = 0 in this ring… Hence inside this ring we have that 8^n = 3^n + 5^n (mod 15) also contradicting the Fermat stuff.

I did some internet searches like ‘Fermat last theorem and divisors of zero’ but weirdly enough nothing popped up. That was weird because I view the depth of the math results related to this divisor of zero as the depth of a bird bath. It is not a deep result or so, just a few centimeters deep. But sometimes just a few centimeters can bring a human mind into another world. For example a long time ago when I still was as green as grass back in the year 1986 I came across the next excercise: Calulate the rest of 103 raised to 103 and divided by 13. I was puzzled, after all 103^103 is a giant number so how can you find the rest after dividing it by 13? But if you give that cute problem a second thought, after all that is also bird bath deep because you can solve it with your human brain…

This post is 11 pictures long, all of the standard size of 550×775 pixels. Because I could not find anything useful about the last Fermat theorem combined with divisors of zero I included a small addendum so all in all this post is 12 pictures long.

After so much Gaussian integer stuff, there is only one addendum about the integers modulo 30. In that ring you can also find some contradictions to the standard way of presenting the last theorem of Fermat.

Ok, if you are still fresh after all that modulo 30 stuff, for reasons of trying to paint an overall picture let me show you a relatively good video on the Kummer stuff. Interesting in this video is that Kummer used the words `Ideal numbers´ and at present stuff like that is known as an ideal. For myself speaking I never use the word ´ideal´ for me these are ´multiplicative attractors´ because if a number of such an ideal multiplies a number outside that ideal, the result is always inside that ideal. Here is a relatively good video:

And now you are at the end of this post. Till updates.