The proof is finished and in the end it went rather different from what I expected before the writing down of this new proof. I hope the main ideas are easy to understand. I formulated the proof with a concrete example; so not two general prime number p and q. But I took p = 5 and q = 7 and as such we are calculating in the ring of integers modulo 35.
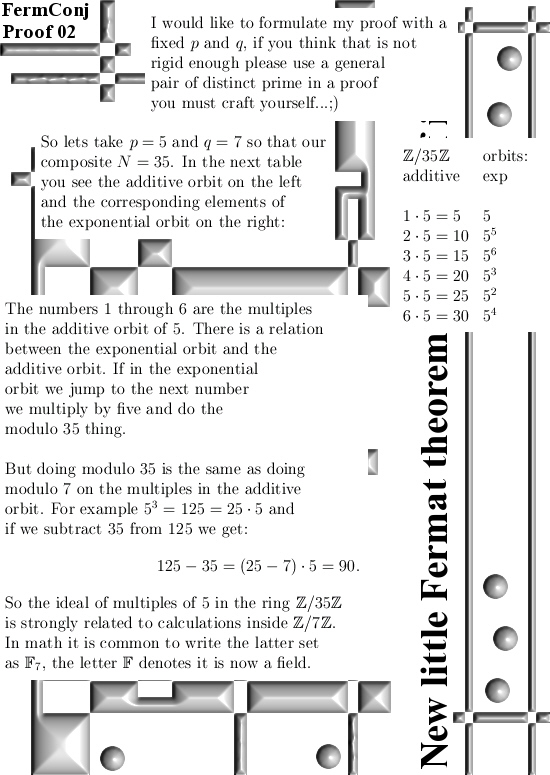
One of the key ideas is that we have so called additive orbits, for example the additive orbit of 5 is the set {0, 5, 10, 15, 20, 25, 30}. The additive orbit are just the multiples of 5, it is handy to view the above set as multiples of five and as such: {0, 5, 2*5, 3*5, 4*5, 6*5}.
On the other hand we have exponential orbits, the exponential orbit of 5 are the powers of 5 like in the next sequence: 5, 5^2, 5^3, 5^4…
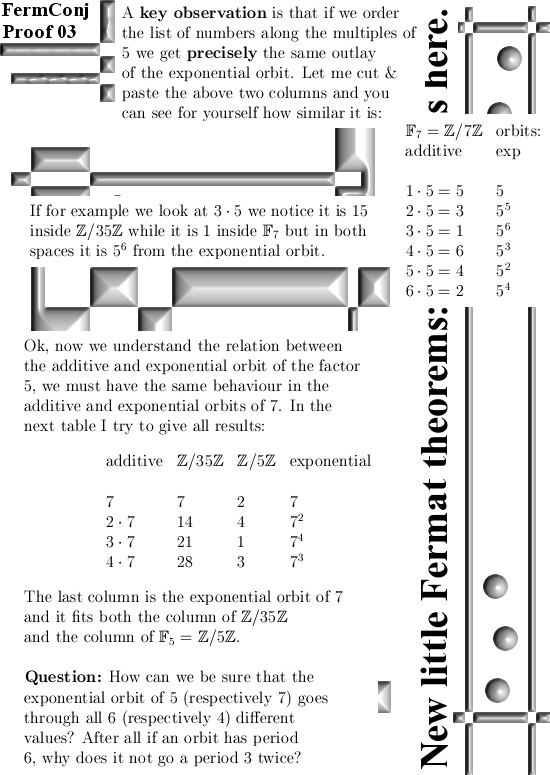
An important observation is that any power of five is also a multiple of five; that means the exponential orbit is inside the additive orbit. For example 5^3 is on the exponential orbit, 5^3 = 125 = 20 modulo 35 = 4* 5.
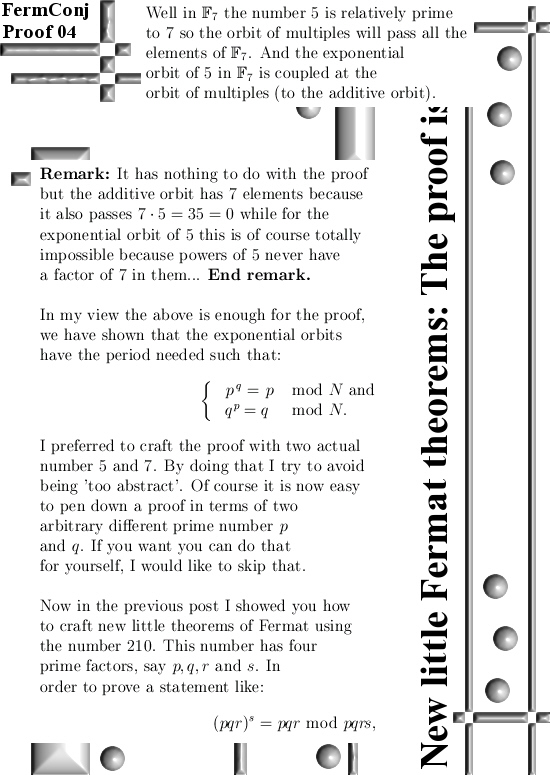
So the number 5^3 from the exponential oribt corresponds to 4*5 on the additive orbit. The goal of the proof is to show that the period of 5 in her exponential oribt is 6 and the period of the exponential orbit of 7 is 4.
That will ensure our new little theorems of Fermat:
p^q = 5^7 = 5 modulo 35 &
q^p = 7^5 = 7 modulo 35.
Remark that powers of 5 can never be a multiple of 35 simply because any power of 5 does not contain a prime factor 7, so not all of the numbers on the additive orbit are allowed.
Another key idea is that if we reduce stuff modulo 35, this is the same as reducing stuff modulo 7 on the multiples of 5. Let me explain: Take the number 50, inside the mod 35 ring this is 15. But 50 = 10* 5 = (10 – 7)*5. I was able to pull that modulo 35 stuff on a ring back to modulo 7 stuff on a field…

Another thing I want to remark is that I formulated these new little theorems of Fermat mostly in prime numbers. That makes them more symmetric like the beautiful pair of equations above, but it can be a bit more general like I showed you in the last post using the number 210 that is made up of four different prime factors. And even that is not needed; prime factors can be double or triple it does not matter. As long as the exponent is a prime number my freshly crafted proof will sail you through all the troubles there are.

In a parallel development I found a perfect math professor. It’s a female and she has an amazing career record: At age three already a Fields medal while before she was nine years of age already the third Abel prize for lifetime achievement math… It is surely amazing…;)

But serious, this post is not that long. Only five pictures and like I said above it is not a ‘most general’ kind of proof but it uses a fixed pair of prime numbers. I think it is better this way because if I would formulate all the stuff in a general setting the only people who could understand such a writing are the ones who already figured the stuff out for themselves… Ok, all pictures are in the usual 550×775 pixelf format so here we go:

We are almost at the end: Let me give you one more example as why only in the exponent you need a prime number. We take the number 8 and raise it to the power 5 and do the reducing modulo thing by 40.
Doing so gives that: 8^5 mod 40 = 32768 mod 40 = 32768 – 819*40 = 8.

Ok, that is what I had to say for this post.