This is not a totally new proof, basically it is already part of the proof in the previous post. But I was able to write it down a bit more compact. It seems that you can skip large parts of the previous proof and still the result is standing. The stuff you can skip is not unimportant or so; it is where I compared those additive and multiplicative orbits and you really need that in order to understand a bit more of what is happening in rings like the integers modulo 35 (or any other composite number of course).
Often I name the ordinary intergers ‘real integers’. I hope that is not confusing, with a real integer I mean whole numbers as they are found on the real line. I do that do make clear the difference with the Gaussian integers as they are found in the diverse complex spaces.

Right now I am already about four months busy with this stuff that all started in January with counter examples to the last theorem of Fermat. All in all I never expected to be able to make an improvement on the little theorem of Fermat. But sometimes the old little theorem gives answers that are indeed correct but still is not that satisfactory. In the old little theorem you take some real integer a, you pick a prime number p that has no common factors with a and you know that in that case:
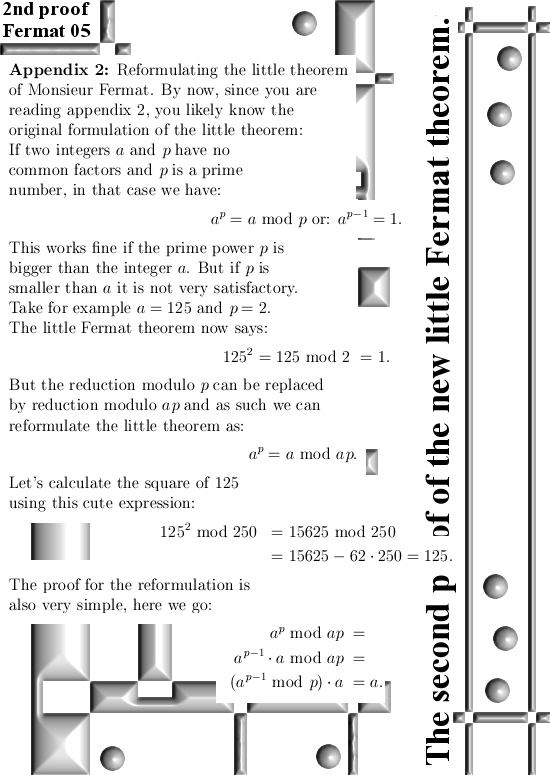
a^p mod p = a. But if the prime number p is relatively small, I mean a is larger, you don’t get back a but a mod p.

This post is short, only three pictures in the usual format of 550×775 pixels but I added two appendices so all in all there are five pictures in this post. In the second appendix I show you that if we square 125, the old little theorem gives back a 1 while my new version of the little theorem nicely gives back 125. Of course there is always much discussion possible of something is ‘better’ or not. It is only in the sense that also with small prime numbers you get back your a I mean it is ‘better’. One thing is clear: it is definitely more beautiful. My favorite formulation stays the formulation with two prime numbers like in:

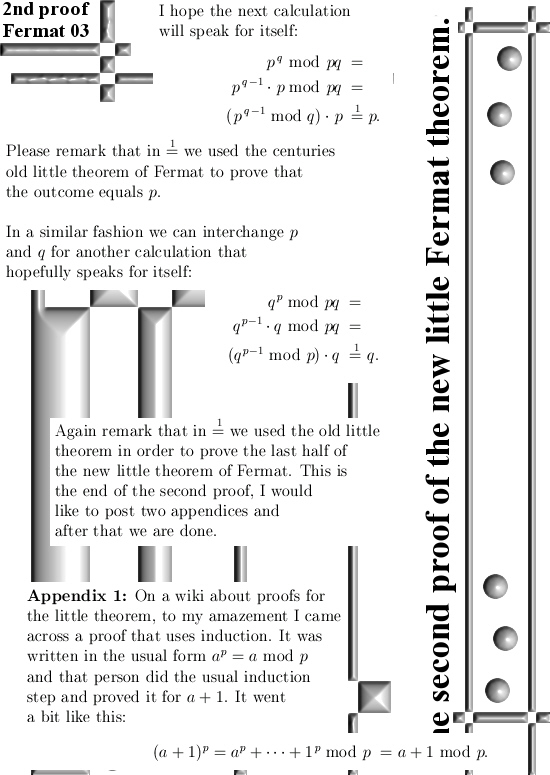
p^q = p mod pq &
q^p = q mod pq.

The symmetry in the pair of equations above is, in my opinion, more beautiful compared to the old version of the little theorem of Fermat. At last I want to remark that I have a thing in common with Monsieur Fermat: math is a hobby for me. Now the old little theorem was improved upon by the professional professor Euler and with a little smile on my face I can say: Hey Euler did you miss the above pair of cute equations?

Yes he did, just like a guy named Einstein never had a fucking clue about electron spin. But likely that is a story for another day, in the meantime we have five pictures with the second proof of the new little theorem. Have fun reading it and never forget: If it is math, sometimes you need a few more days to figure it all out!

In a wiki upon proofs of the little Fermat theorem you can find that one of the authors did indeed find a counter example to the last theorem of Fermat. You can find it under ´Multinomial proofs´in the next link:
Proofs of Fermat´s little theorem. Link used:
https://en.wikipedia.org/wiki/Proofs_of_Fermat%27s_little_theorem

Ok, that was it for this post. Thanks for your attention.