Once more I estimated my writer skills too positive; I thought it takes only 7 to 8 pictures to pen down all counter examples to the last theorem of Pierre de Fermat. But I had to stop because this post already is 10 pictures long. I organized the stuff around so called ‘levels’ so we have Level zero counter examples (the most basic ones) to level four counter examples (the most complicated ones) to the last theorem of Fermat.
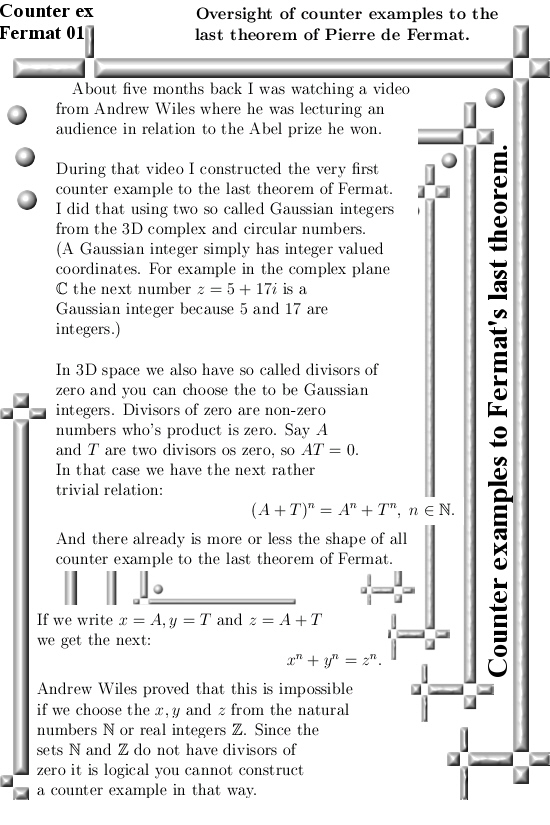
We are now almost half a year further down the timeline since I looked at that video of Andrew Wiles and while looking at that video I already constructed the first counter example to the last theorem. And now we are about half a year further I have arrived at the conclusion that inside the math community there are no accepted counter examples known or discussed or whatever what they do with it. So I am not expecting my counter examples will find the place they more or less should have, acting as an adult is never within reach of the math professors.
Likely if you (as a math student or so) show these counter examples to one of your math professors, it might be it gets instantly rejected because ‘this is modulo calculus’ and has ‘nothing to do with the last theorem’. But in a pdf from Gerhard Frey (the guy from the Frey elleptic curve) I came across the next hilarious screen shot:

Of course people like Andrew Wiles or Gerhard Frey will never ever react; they are perfumed princes and those high nobility people do not mingle with the plebs, farmers and peasants.
Does the absence of counter examples on the internet indicate that the math hot shots also have an absence of counter examples in their holy brain tissue? It looks like it, of course it is very well possible plenty of people found those counter examples in the past but they got only a fresh dose of what I experience for say 30 years: You get one 100% neglected all of the time. After all if you are an outsider like me, the collective of math professionals acts very much like a secretive incest club. Those kind of clubs are not very open to communication with the outside world. Not that math professors are pedophiles but the behavior is keeping things inside is very much the same.
An interesting question is if perfumed prince Andrew Wiles knows these counter examples. My guesstimate is no. And why is that? After the pictures of the main post I will show you that video from the Abel prize lecture and there you can see Andrew formulating the last theorem of Pierre de Fermat. He does it more or less in the following manner:
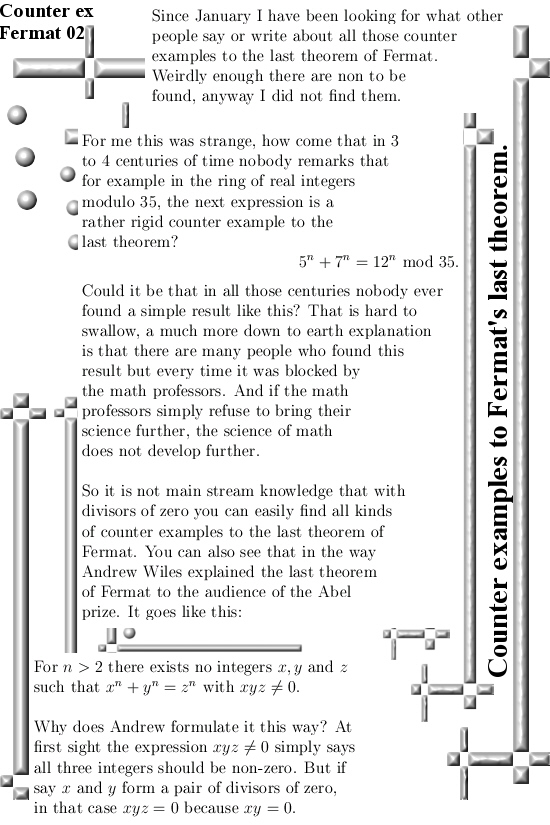
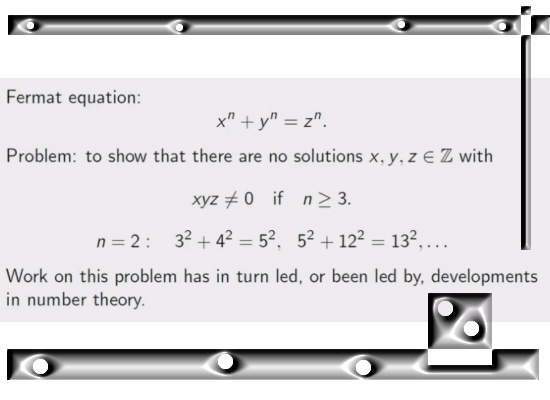
For integers x, y and z with xyz not equal to 0, it is impossible that
x^n + y^n = z^n. This for integers n > 2.
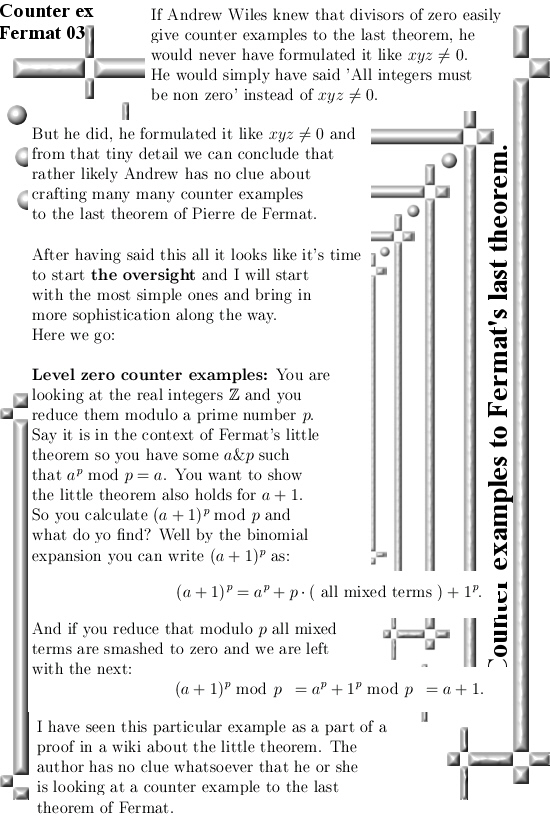
If Andrew would have knowledge in his brain about these counter examples likely he would have formulated it like this:
For positive non-zero integers x, y and z, it is impossible that
x^n + y^n = z^n. This for integers n > 2.
All counter examples I found in the last five months have the property that xyz = 0 because say the x and the y form a pair of so called divisors of zero. (The level zero counter examples do not have this property, but I did not find the level zero examples myself…) Now it could be that Andrew is as evil as he is smart and formulated it with the xyz not zero way in order to subtlelly exclude my wild math adventures. But in reality it is much more likely that Andrew is just another perfumed Princeton professor. So my estimate is and stays that Andrew is not aware of the rather simple counter examples to the last theorem of Pierre de Fermat as found below…
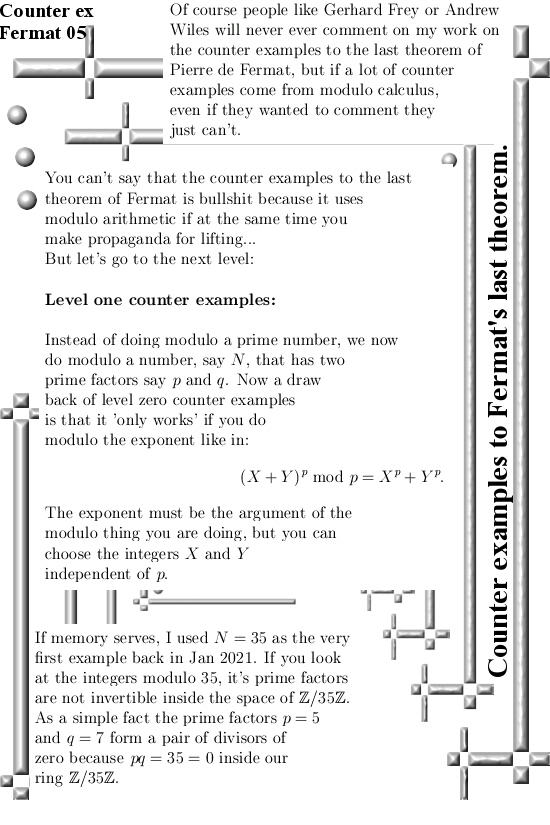
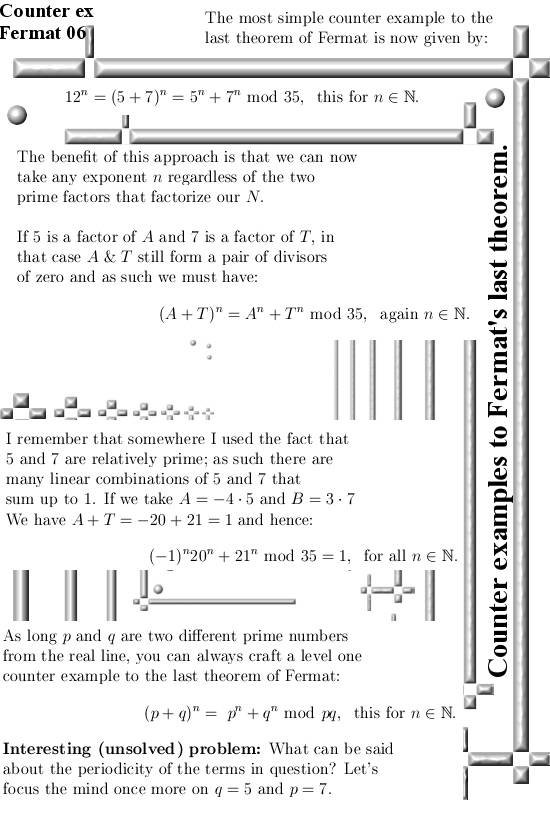
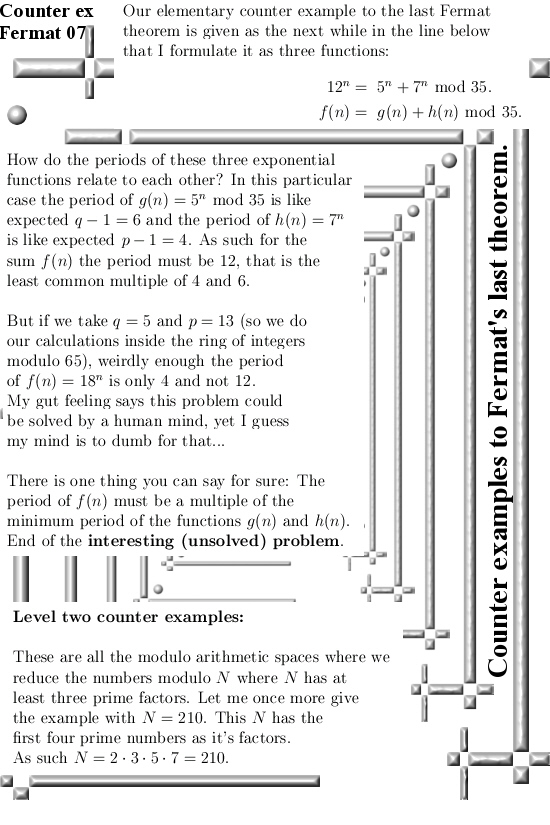
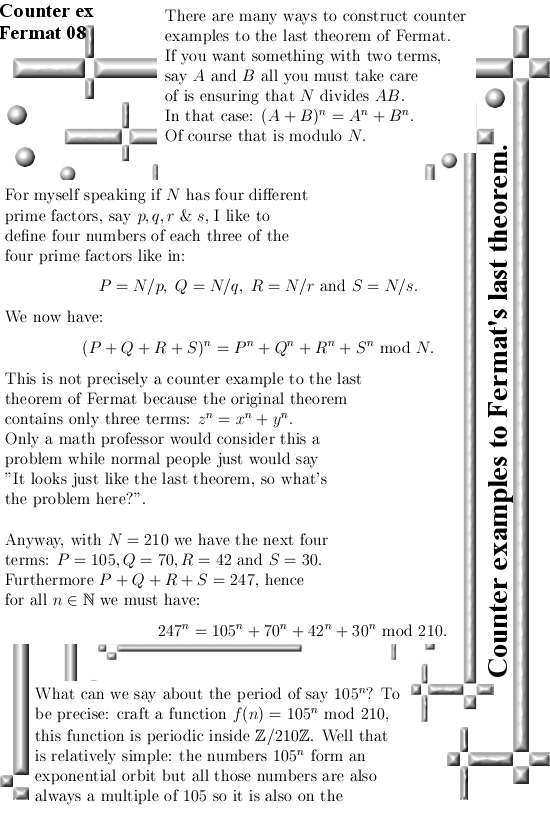
Ok, this post is 10 pictures long and it only contains the stuff found that is based on modular arithmetic. In my view it is all rather basic and as such should explain itself. The main basis is that ‘divisor of zero’ stuff where two non zero numbers multiply to zero. For example if we take the real integers modulo 35, in that case if we multiply 5*7 we get 0 because 35 = 0 inside the ring of integers modulo 35. It’s all so basic that it makes you wonder once more: why is this not inside the math classes on universities? But for that answer you must ask the perfumed professors and the perfumed professors never answer because they are much more busy of getting a fresh stack of perfume. That’s life…
Have fun reading it

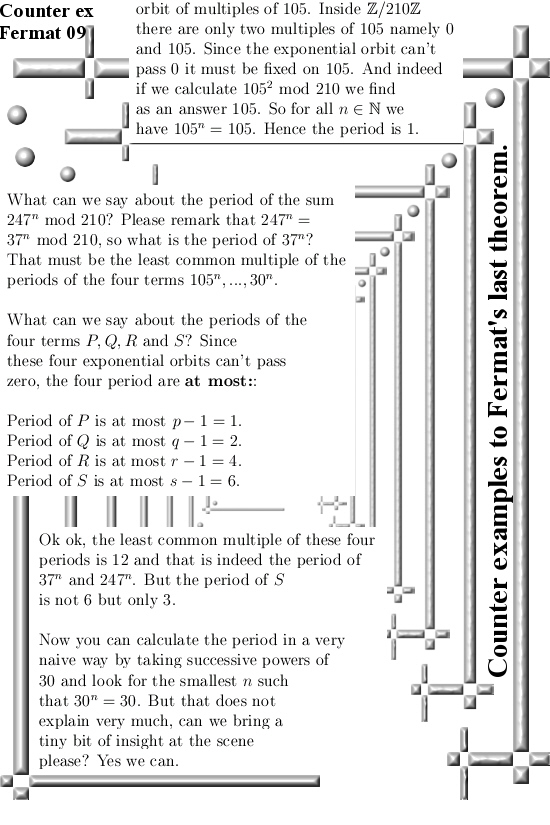
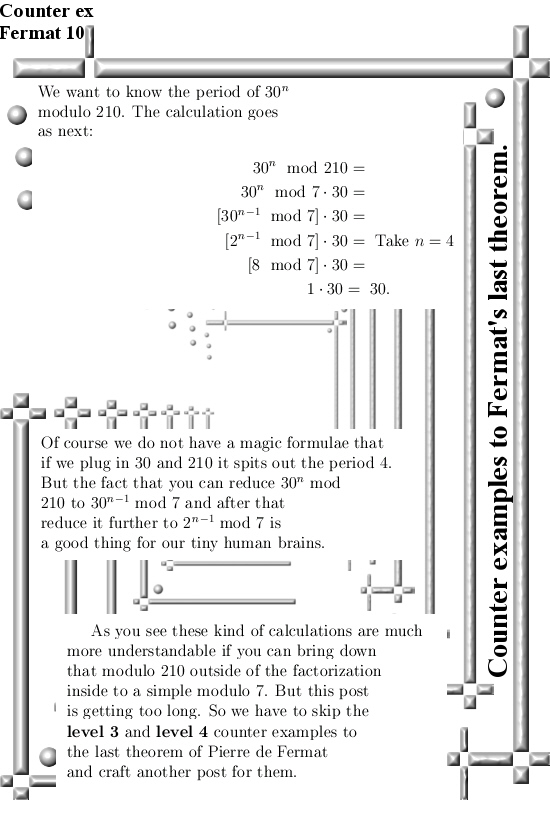
For me the only thing I learned while writing this post is that you can use that simple rule for writing 30^n mod 210 in stuff mod 7. It is the same kind of calculation as in the modified little theorem of Fermat and this is also a detail I could not find on the internet.
Oh yes now I am in a laughing mood: Can we prove the perfumed professors are indeed perfumed overpaid people? Yes we can: They will also not react of the modified or improved little theorem of Fermat. We will need a few years of time for that, but why should they react?
Ok, I promised you the video of a Princeton perfumed prince named Andrew Wiles, here it is and please pay attention to the way he formulates the present day formulation of the last theorem of Pierre de Fermat:
At only one minute into the video we observe that likely Andrew has no clue whatsoever about the above counter examples to the last theorem of Pierre de Fermat:
End of this post. Thanks for your long lasting interest in these details on the last theorem of Pierre de Fermat.