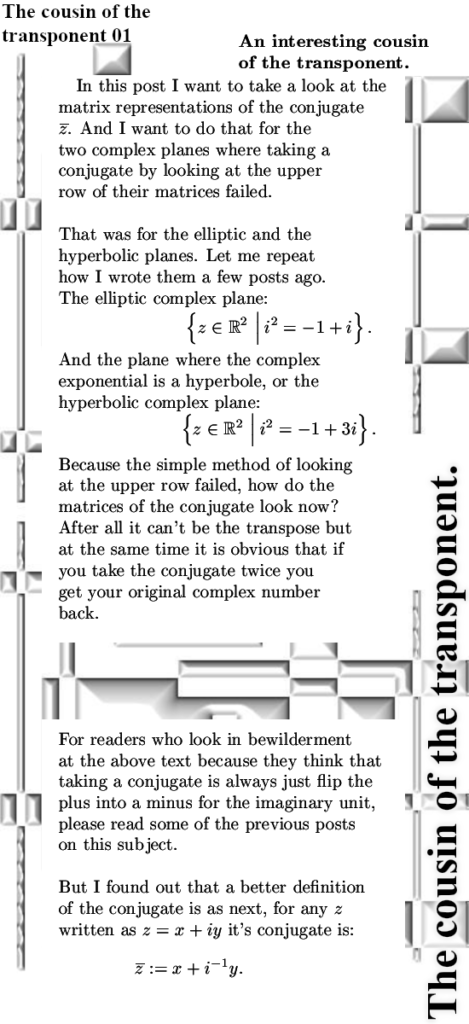
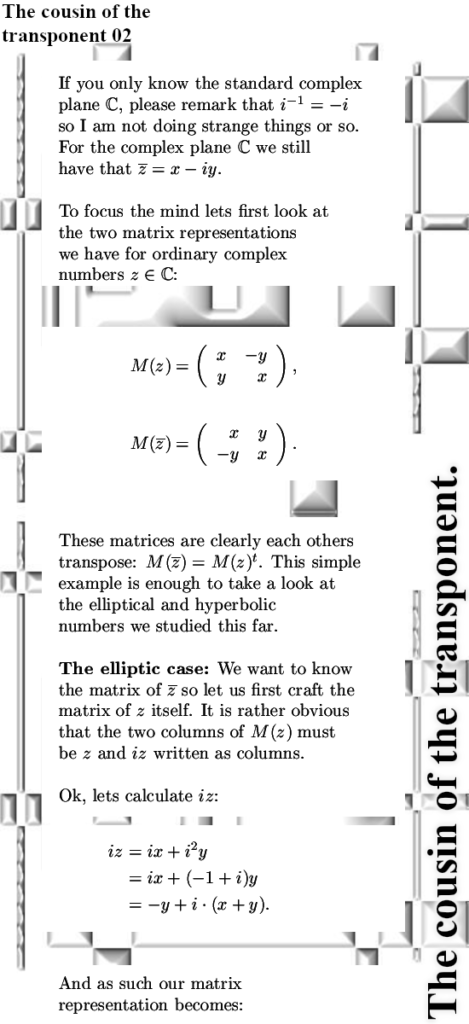
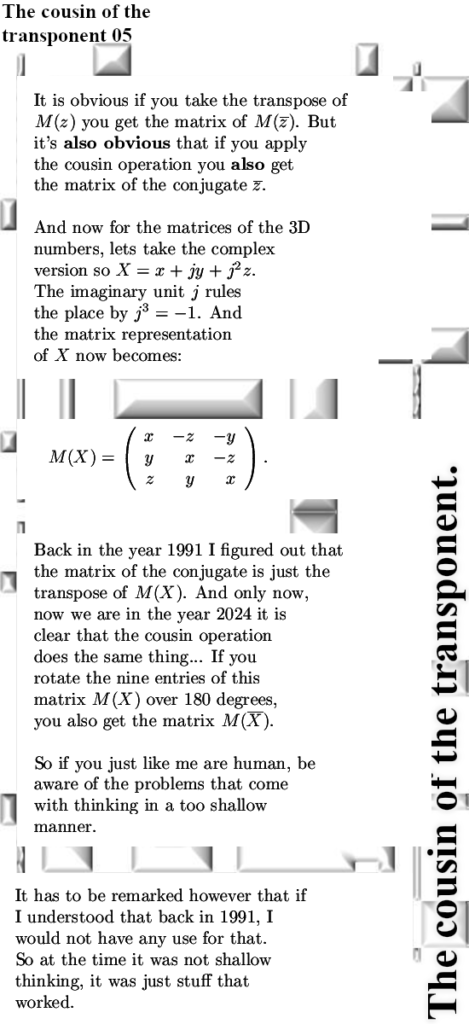
Likely in the year 1991 I had figured out that the conjugate of a 3D complex number could be found in the upper row of it’s matrix representation. As such the matrix representation of a conjugate 3D number was just the transpose of the original matrix representation. Just like we have for ordinary complex numbers from the complex plane. And this transpose detail also showed that if you take the conjugate twice you end where you started from. Math people would say if you do it twice, that is the identity operation.
But for the two 2D multiplications we have been looking at in the last couple of months, the method of taking the upper row as a conjugate did not work. I had to do a bit of rethinking and it was not that hard to find a better way of defining the conjugate that worked on all spaces under study since the year 1991. And that method is replace all imaginary units by their inverse.
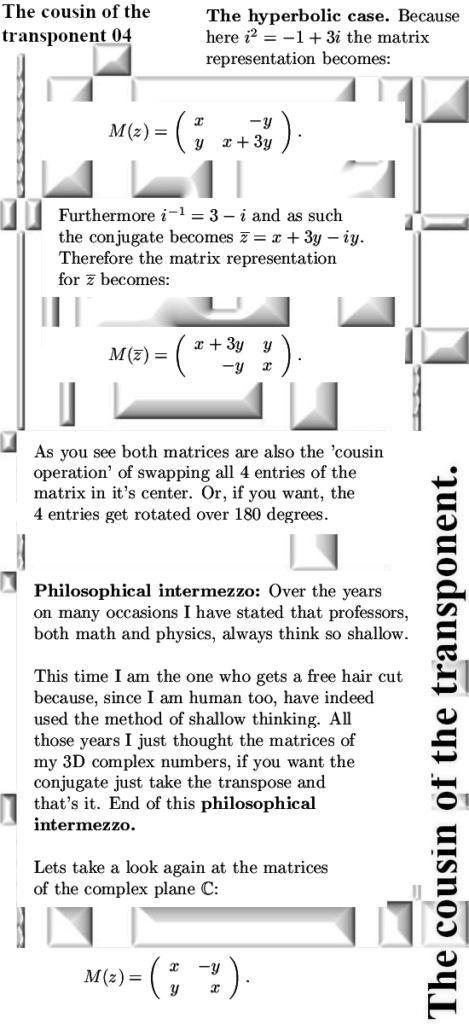
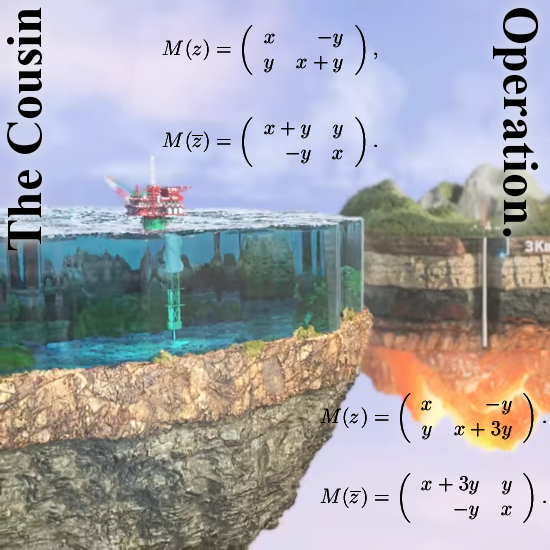
As such we found the conjugate on 2D spaces like the elliptical and hyperbolic complex planes. And the product of a 2D complex number z with it’s conjugate nicely gives the determinant of the matrix representation. And if you look where this determinant equals one, that nicely gives the complex exponentials on these two spaces: an ellipse and a hyperbole.
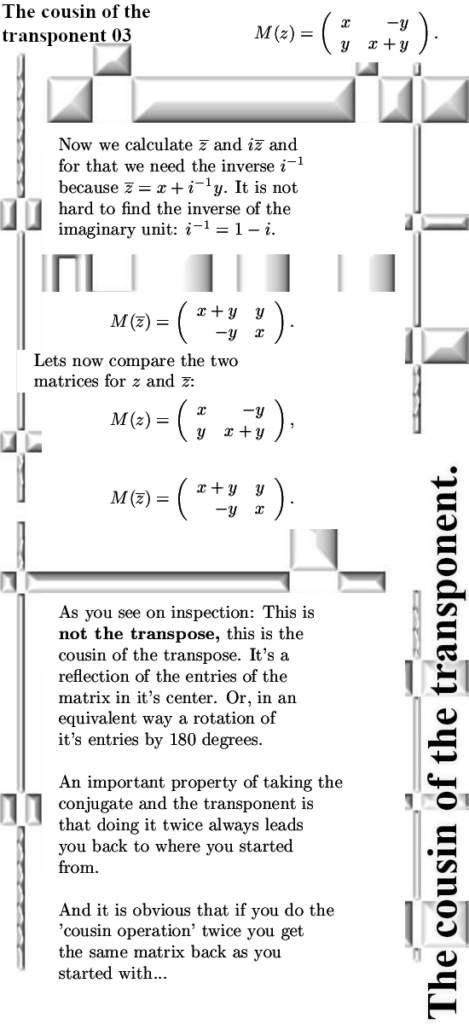
Now when I was writing the last math post (that is two posts back because the previous post was about magnetism) I wondered what the matrix representation of the conjugate was on these two complex planes. It could not be the transpose because the conjugates were not the upper rows. And I was curious what it was, it it’s not the transpose what is it? It had to be something that if you do it twice, you do the identity operation…

All in all in this post the math is not very deep or complicated but you must know how te make the conjugate on say the elliptic complex plane. On this plane the imaginary unit i rules the multiplication by i ^2 = -1 + i. So you must be able to find the inverse of the imaginary unit i in order to craft the conjugate. On top of that you must be able to make a matrix representation of this particular conjugate. If you think you can do that or if you don’t do it yourself you will understand how it all works, this post will be an easy read for you.

It turns out that the matrices of the conjugate are not the transpose where you flip all entries of the matrix into the main diagonal. No, these matrix representation have all their entries mirrored in the center of the matrix or equivalently they have all their entries rotated by 180 degrees. That is the main result of this post.

So that’s why I named it the “Cousin of the transponent” although I have to admit that this is a lousy name just like the physics people have with naming the magnetic properties of the electron as “spin”. That’s just a stupid thing to do and that’s why we still don’t have quantum computers.

Enough intro talk done, the post is five pictures long and each picture is 550×1200 pixels. Have fun reading it.

That was it for this post, one more picture is left to see and that is how I showed it on the other website. Here it is:

Ok, this is really the end of this post. Thanks for your attention and may be see you in another post of this website upon complex numbers.