It’s about time to write this post because the pictures were finished a few days back but I was a bit lazy in the meantime. In this post I only evaluate two line integrals both in some way related to the famous number alpha. And we do it only on the 3D space, there are much more numbers alpha on other spaces but we just do the complex and the circular multiplication in three dimensions.
In this post when it comes to the number alpha we mostly need the one property that alpha is it’s own square. Therefore you can break down all powers of alpha into alpha itself, this is very handy when for example you use a power series. As always X denotes a 3D number and one of the integrals we will look at is the exponential of alpha times X:
exp(alpha * X).
Why do we do this? Well try to find a primitive of the above exponential, that is a bit hard because another property of alpha is that it has no inverse and as such you can’t divide by alpha and so what to do?
The second example is integration of the most standard exponential exp(X) along the main axis of non-invertibles: All real multiples of the number alpha. For me this was a surprising result because it all becomes so much more simple. All in all this post is five pictures long but it is in the 550×1500 pixel size so they are relatively long. Ok that is all I had to say and let me now hang in the five pictures:
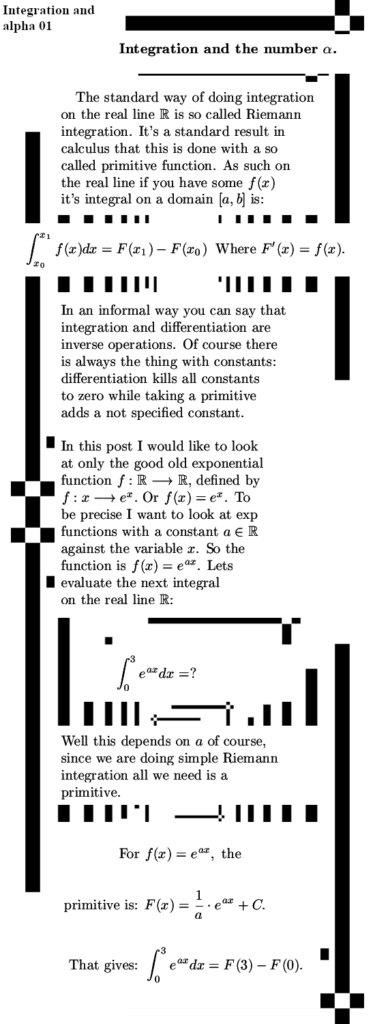
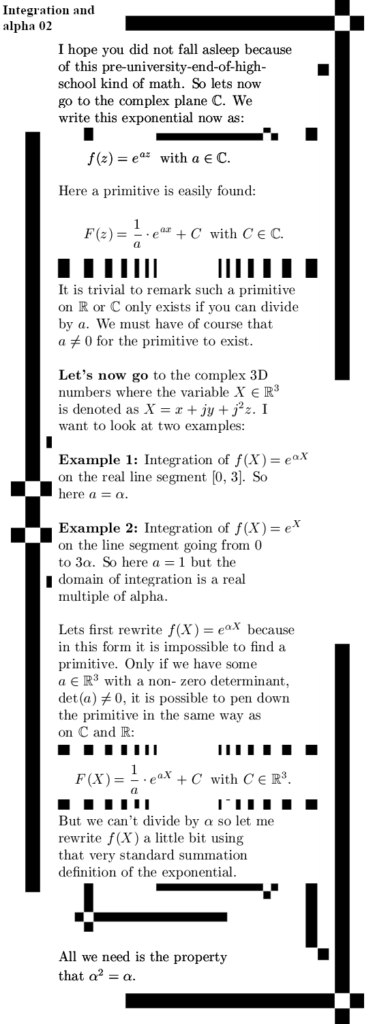
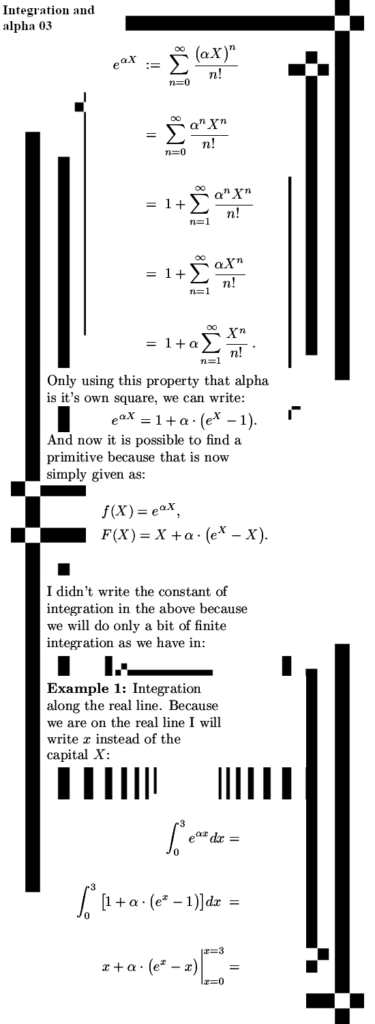

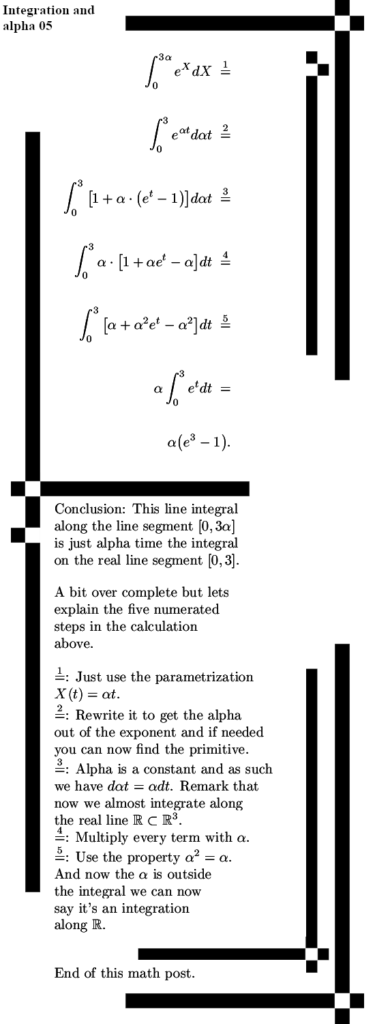
That was it for this post, thanks for your attention and may be see you in a future post.